Surface Areas and Volumes Class 10 MCQ – Maths Class 10 MCQ Online Test are covered in this Article. Surface Areas and Volumes Class 10 MCQ Test contains 30 questions. Answers to MCQ on Surface Areas and Volumes Class 10 are available after clicking on the answer. MCQ Questions for Class 10 with Answers have been made for Class 10 students to help check the concept you have learnt from detailed classroom sessions and application of your knowledge.
| Board | CBSE |
| Textbook | Maths (NCERT) |
| Class | Class 10 |
| Chapter | Chapter 13 Surface Areas and Volumes |
| Category | MCQ Questions for Class 10 Maths with Answers |
Surface Areas and Volumes Class 10 MCQ
1.How many shapes are there in the figure
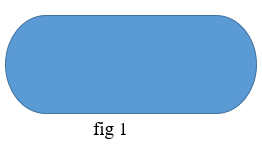
(a) 1
(b) 2
(c) 3
(d) 4
Answer
Answer: (c) 3
Explanation:
hemisphere +cylinder+ hemisphere
2. Find the TSA of fig1

(a) 2πrh
(b) 2πr (r+ h)
(c) πr2h + 2π
(d) 2πr(2r+h)
Answer
Answer: (d) 2πr(2r+h)
Explanation:
TSA of given figure = CSA of cylinder+ 2×CSA of Hemisphere
= 2πrh + 2×(2πr²) = 2πrh + 4πr²
= 2πr (h+2r)
3. Volume of cone is…………
(a) 1/3πr2h
(b) 4/3πr2h
(c) 2πr2h
(d) 1/3πrh
Answer
Answer: (a) 1/3πr2h
4. Find TSA of a pencil sharpened at both ends.
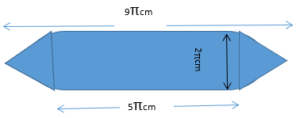
(a) 2πr ×l
(b) 2π (r + l)
(c) 2π2(2 +√5)
(d) 2π3 (2 + √5)
Answer
Answer: (d) 2π3 (2 + √5)
Explanation:
TSA = CSA of Cylinder + 2× (CSA of cone)
= 2πrh + 2× (πrl) = 2πr (h +l)
In cylinder, r = 2π/2 =πcm, h = 5πcm
In cone, r = 2π/2= πcm,
h = 2πcm
(Total height – h of cylinder=9π -5π = 4π, h of one cone =4π/2 = 2πcm)
l = √ r2 + h2
= √ (π)2 + (2π)2
= √ π2 + 4π2 = √ 5π2 = π√5cm
TSA = 2πr (h +l)
= 2πx π (2π +π√5)
= 2π3 (2 + √5)
5.Name the figure and find the area.
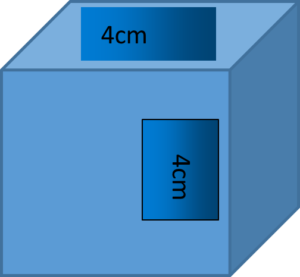
(a) Cuboid, 96cm2
(b) Cube, 96cm2
(c) Cuboid, 96cm3
(d) Cube, 69cm3
Answer
Answer: (b) Cube, 96cm2
Explanation:
The given figure is Cube.
Area of Cube = 6 × (side)2
= 6 × (4)2
= 96cm2
6. Name the shapes obtained by slicing the cone by a plane parallel to base.
(a) Cylinder
(b) Cone + cylinder
(c) Cone + frustum
(d) Cylinder+ frustum
Answer
Answer: (c) Cone + frustum
Explanation:
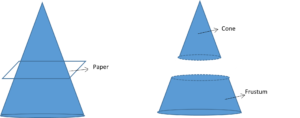
7. If one solid is converted into another solid, then the volume of the new shape will
(a) Increase
(b) Decrease
(c) Same
(d) Doubled
Answer
Answer: (c) Same
Explanation: When one solid is converted into another solid, the volume of the new shape will remain same.
Surface Areas and Volumes Class 10 MCQ
8. A metallic hollow cylinder of height 6cm and internal and external diameters 6cm and 10cm respectively, is melted and recast into the form of a cone with base diameter 8cm. The height of the cone is
(a) 10cm
(b) 18cm
(c) 12cm
(d) 16cm
Answer
Answer: (b) 18cm
Explanation:
When one solid is converted into another solid, the volume of the new shape will remain same.
Volume of cylinder = volume of cone
Π(R2 – r2)h = 1/3 Πr2h
Π(52 – 32) × 6 = 1/3 × Π × 42x h
Π × 16 × 6 = 1/3 × Π × 16 x h
h = 18cm
9. A room is in a shape of cuboid measuring 20m x 10m x 5m. Find the area of the room. If cost of painting is 15/m2, find the cost paid.
(a) 500m2, Rs5000
(b) 600m2, Rs 7500
(c) 700m2, Rs 7000
(d) 700m2, Rs 7500
Answer
Answer: (d) 700m2, Rs 7500
Explanation:
Surface area of room = TSA of cuboid = 2 (lb + bh + hl)
= 2 (20×10 + 10×5 + 5×20)
= 2 (200 + 50 + 100)
= 2 × 350 = 700m2
For painting the room, walls and ceiling has to be painted, floor should be excluded from the area.
Area of painting the room = TSA – Area of Floor
= 700 – l × b
= 700 -200 = 500m2
Cost of painting = 500 x 15 = Rs7500
10. Find the volume of cone, if radius is doubled and height is halved.
(a) V1 = 2V2
(b) V2 = 2V1
(c) V1 = 2/3V2
(d) V2 = 2/3V1
Answer
Answer: (b) V2 = 2V1
Explanation:
Vol of cone = 1/3 × Π × r2× h
Here, r = 2r; h = h/2
Vol of cone = 1/3 x Π × (2r)2× h/2
= 1/3 x Π × 4r2× h/2
= 2/3 × Π × r2× h
11. A solid cone of radius r and height h is placed below an another cone of radius r and height 2h in a such a way that tip of the below placed cone is pointing downward. Find the TSA.
(a) π × r × [ (√ r2 + h2)
(b) π × r × (√ r2 + 4h2)
(c) π × r × [ √ h2 + 4h2)]
(d) π × r × [ (√ r2 + h2) + (√ r2 + 4h2)]
Answer
Answer: (d) π × r × [ (√ r2 + h2) + (√ r2 + 4h2)]
Explanation:
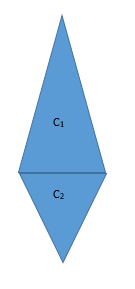
TSA = CSA of C1 + CSA of C1
= πr1l1 + πr2l2
= π × r × √ r2 + h2 + π × r × √ r2 + 4h2
= π × r × [(√ r2 + h2) + (√ r2 + 4h2)]
12. Find the radii of a sphere, when 10 solid spheres of same radii R are being made by melting a cone of radius 3cm and height 12cm.
(a) 2cm
(b) 3cm
(c) 4cm
(d) 5cm
Answer
Answer: (b) 3cm
Explanation:
When one solid is converted into another solid, the volume of the new shape will remain same.
Volume of sphere = volume of cone
4/3 π R3 = 1/3 πr2h
4 × π × R3 = π × 3×3 × 12
R3 = 3×3×3
R = 3cm
13. Volume of two hemispheres are in a ratio of 64:125. Find the ratio of its surface areas.
(a) 4/5
(b) 16/25
(c) 25/16
(d) None of the above
Answer
Answer: (b) 16/25
Explanation:
V1 / V2 = 64 /125
2/3 π r13 / 2/3 π r23
= 64 / 125
(r1 / r2)3 = 64/125
r1 / r2 = 4/5
S1 / S2 = 3π r12 / 3π r22
= (r1 / r2)2
= (4/5)2 = 16/25
14. The diameters of two circular ends of a tub of height 21cm are 20cm and 30cm. Find the volume of tub. (π = 22/7)
(a) 30000 cm3
(b) 45000 cm3
(c) 56500 cm3
(d) 10450cm3
Answer
Answer: (d) 10450cm3
Explanation:
A tub is in a shape of frustum.
So, vol of tub = vol of frustum = 1/3 π h ( R2 + r2 + Rr)
= 1/3 × 22/7 × 21 × (100 + 225 + 150)
= 22 ×475 = 10,450cm³
15. Find TSA of given figure
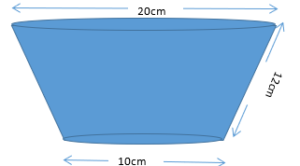
(a) 960 cm2
(b) 958.6 cm2
(c) 957.04 cm2
(d) 958 cm2
Answer
Answer: (b) 958.6 cm2
Explanation:
Given shape is frustum
R = 20/2 = 10cm, r = 10/2 =5cm
TSA = πR2 + πr2 + π (R + r) l
= π {102 + 52 + (10 + 5) ×12}
= 22/7 {100 + 25 + 180}
= 22/7 × 305
= 958.57 cm2
= 958.6 cm2
16.Find the height of cuboid if length and breadth of it are 6cm and 5cm respectively, given that the lateral surface area of cuboid is 110cm2.
(a) 4cm
(b) 7cm
(c) 5cm
(d) 8cm
Answer
Answer: (c) 5cm
Explanation:
CSA of cuboid = 2 (lh + bh)
110 = 2 (6 × h + 5 × h) = 2 (11h)
h = 110/22 = 5cm
Surface Areas and Volumes Class 10 MCQ
17. Find the area of shaded portion, where both the cones are same in size.
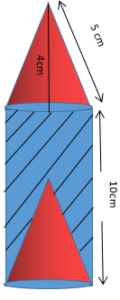
(a) 100cm2
(b) 98.5cm2
(c) 95cm2
(d) 141.4cm2
Answer
Answer: (d) 141.4cm2
Explanation:
In cone, l= 5cm, h = 4cm, r =?
l2 = r2 + h2
r2 = l2 – h2 = 25 – 16 = 9
r = 3cm
In cylinder, r =3cm, h = 10cm
Area of shaded portion = CSA of cylinder – CSA of cone
= 2πrh – πrl
= 2π × 3× 10 – π× 3 × 5
= 22/7 × 3 (20-5)
= 22/7 × 3 × 15
= 141.4cm2
18. Find the volume of cube if perimeter is 12x.
(a) 8x3
(b) 6x3
(c) 6x2
(d) x3
Answer
Answer: (d) x3
Explanation:
Perimeter = 12a = 12x
a = x = Side of cube
Volume of cube = a3
= (x)3 = x3
19. The ratio of radii and height of two cylinders are 1:1 and 3:2 respectively, find the ratio of their curved surface areas.
(a) 2:3
(b) 1:3
(c) 3:2
(d) 3:1
Answer
Answer: (c) 3:2
Explanation:
S1 / S2 = 2πr1h1 / 2πr2h2
= ( r1/r2) (h1/h2)
= ( 1/1)x (3/2) = 3/2
20. Find the volume of given figure
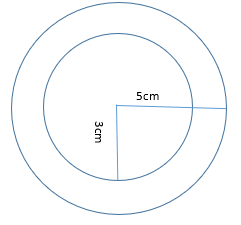
(a) 550 cm2
(b) 500 cm2
(c) 410.0cm2
(d) 410.7cm2
Answer
Answer: (d) 410.7cm2
Explanation:
Shape is spherical shell
Volume = 4/3 π (R3 – r3)
= 4/3 x 22/7 x (53 – 33)
= 4/3 x 22/7 x (125- 27)
= 4/3 x 22/7 x 98
= 410.7 cm2
21. A boy, Kritagya likes an icecream. He wants to paint this picture, the cone with pink colour and cream with yellow colour. Find the yellow colour area to be painted.
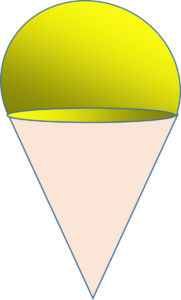
(a) 2πr2
(b) 3πr2
(c) 4πr2
(d) None of the above
Answer
Answer: (b) 3πr2
Explanation:
Area of yellow colour = TSA of hemisphere
= 3πr2
22. Find the volume of cylinder, if the curved surface area of cylinder is 1936cm2 and height is 22cm.
(a) 4415π cm3
(b) 4415π cm3
(c) 4315π cm3
(d) 4312π cm3
Answer
Answer: (d) 4312π cm3
Explanation:
CSA of cylinder = 2πrh
1936 = 2 π × r × 22
r = 88 / 2π = 14cm
Volume of cylinder = πr2h
= π × 14 × 14 × 22 = 4312π cm3
23. Aarav reshapes a hemisphere of volume 36πr3 into a sphere. Find the ratio of their radius.
(a) 3:1
(b) 4:3
(c) 2:3
(d) 3:2
Answer
Answer: (a) 3:1
Explanation:
Volume of hemisphere = Volume of Sphere
36πr3 = 4/3 × πr3
36πr13 = 4/3 × πr23
( r1/r2)3 = 27/1
r1/r2 = 3/1
24. Find the CSA of a coconut shell.
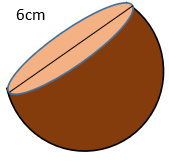
(a) 3π
(b) 15π
(c) 18π
(d) 21π
Answer
Answer: (c) 18π
Explanation:
CSA of a coconut shell = 2πr2 ;( r = 6/2)
= 2 π × 3×3 = 18π
Surface Areas and Volumes Class 10 MCQ
25. A mild steel wire of length 1m is beaten to form a sheet of 11×15×20 m. Find the thickness of wire.
(a) 42m
(b) 10√42 m
(c) 5√42 cm
(d) 5cm
Answer
Answer: (b) 10√42 m
Explanation:
Vol of wire = Vol of sheet
(Volume of cylinder = Volume of cuboid)
Length of wire is height of cylinder = 720m
πr2h = l×b×h
Π × r2 × 1 = 11 × 15 × 20
r2 = 11 × 15 × 20 × 7/ 22 = 1050
r = √1050 = 5√42 m
Therefore, thickness of wire 2 × 5√42 = 10√42
26. TSA of right circular cone
(a) πrl
(b) πr2 (r +h)
(c) πr (l + r)
(d) πr2h
Answer
Answer: (c) πr (l + r)
27. Find the diagonal of a cube with side 4cm.
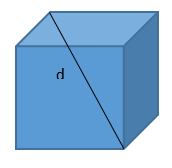
(a) 12cm
(b) 3√4 cm
(c) √3 cm
(d) 4√3 cm
Answer
Answer: (d) 4√3 cm
d = √3 x side = 4√3 cm
28. Find the radius of sphere if volume is 972π.
(a) 9
(b) 7
(c) 5
(d) 4
Answer
Answer: (a) 9
Explanation:
Vol of sphere = 4/3 πr3
972π = 4/3 π × r3
r3 = 972 × 3/4 = 729
r = 9
29. There is a solid cylinder of height 3.6cm and radius 2.1cm .A spherical cavity is hollowed out from cylinder . Find the volume of remaining solid.
(a) 11 cm3
(b) 11.088cm3
(c) 12.08 cm3
(d) 12.088cm3
Answer
Answer: (b) 11.088cm3
Explanation:
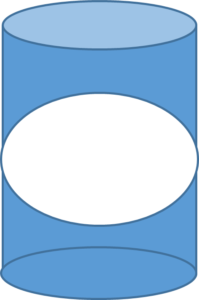
Volume of remaining solid
= Vol of cylinder – Vol of sphere
= πr2h – 4/3 πr3
Radius of cylinder = radius of sphere = 2.1cm
Volume of remaining solid = πr2 (h – 4/3 r)
= 22/7 × 2.1 × 2.1 × (3.6 – 4/3 × 2.1)
= 22 × 0.3 × 2.1 × (3.6 – 2.8)
= 22 × 0.3 × 2.1 × 0.8
= 11.088 cm3
30. Find the ratio of TSA and CSA of hemisphere.
(a) 1:2
(b) 1:3
(c) 1:4
(d) 3:2
Answer
Answer: (d) 3:2
Explanation:
TSA of hemisphere = 3 πr2
CSA of hemisphere = 2 πr2
TSA / CSA = 3 πr2 / 2 πr2 = 3: 2
MCQ Questions for Class 10 Maths
- Real Numbers Class 10 MCQ with Answers
- Polynomials Class 10 MCQ with Answers
- Pair of Linear Equations in Two Variables
- MCQ Questions on Quadratic Equations for Class 10
- Arithmetic Progressions
- Triangles Class 10 MCQ with Answers
- Coordinate Geometry
- Introduction to Trignometry
- Some Applications of Trigonometry Class 10 MCQ with Answers
- Circles Class 10 MCQ with Answers
- Constructions Class 10 MCQ with Answers
- Areas Related to Circles
- Surface Areas and Volumes
- Statistics Class 10 MCQ with Answers
- Probability Class 10 MCQ
Frequently Asked Questions on Surface Areas and Volumes Class 10 MCQ
1. Are these MCQ on Surface Areas and Volumes Class 10 are based on 2021-22 CBSE Syllabus?
Yes . There are 30 MCQ’s on this Chapter in this blog.
2. Are you giving all the chapters of Maths Class 10 MCQs with Answers which are given in CBSE syllabus for 2021-22 ?
Yes, we are providing all the chapters of Maths Class 10 MCQs with Answers.