Pair of Linear Equations in Two Variables Class 10 MCQ with Answers

Pair of Linear Equations in Two Variables Class 10 MCQ with Answers are covered in this Article. Pair of Linear Equations in Two Variables Class 10 MCQ Test contains 25 questions. Answers to MCQs on Pair of Linear Equations in Two Variables Class 10 Maths are available after clicking on the answer. MCQ Questions for Class 10 Maths with Answers have been made for Class 10 students to help check the concept you have learnt from detailed classroom sessions and application of your knowledge.

Board	CBSE
Textbook	Maths (NCERT)
Class	Class 10
Chapter	Chapter 3 Pair of Linear Equations in Two Variables
Category	MCQ Questions for Class 10 Maths with Answers

Pair of Linear Equations in Two Variables Class 10 MCQ with Answers

1. A pair of linear equation a₁ x+ yb₁+ c₁=0, a₂ x+ b₂y+ c₂=0, is said to consistent, if

(a)  a₁ /a₂  ≠ b₁ /b₂

(b) a₁ /a₂= b₁ /b₂

(c) a₁ /a₂ = b₁ /b₂ ≠ c₁ /c₂

(d) None

Answer

Answer: (a)  a₁ /a₂  ≠ b₁ /b₂

Explanation : If a₁ /a₂  ≠ b₁ /b₂, then the pair of linear equations is consistent.

---

 

2. A pair of linear equations which has no solution, is called

(a)consistent

(b) Inconsistent

(c) dependent and consistent

(d) dependent and inconsistent

Answer

Answer: (b) Inconsistent

Explanation: A pair of linear equations which has no solution, is called an inconsistent pair of linear equations.

---

 

3. 9√5 x -√3 y = 10 and  9√5 x – √27 y =0, then value of x and y is

(a)x=√5/3 , y = 5√3/3

(b) x =5√3/3  , y = √3/3

(c) x =5√5/3  and y = 5/3

(d) None of these

Answer

Answer: (a)x=√5/3 , y = 5√3/3

Explanation: 9√5x -√3 y = 10
9√5x -10=√3 y

and y = (9√5x -10)/(√3)

Substituting value of y in 9√5x -√27 y =0

9√5x -√27((9√5x -10)/(√3)) =0

9√5x -3√3((9√5x -10)/(√3)) =0

9√5x – 27√5x + 30 =0

18√5x = 30

x = 30/(18√5) = (√5)/3

and y = (9√5x -10)/(√3)

= (9√5(√5/3) -10)/(√3)

= (15 -10)/(√3)

= 5/(√3) = (5√3)/3

---

 

4.  If in the equation 5x+2y=7 the value of x is 1, then value of y is

(a)-1

(b)0

(c)1

(d)2

Answer

Answer: (c)1

Explanation: 5x+2y=7
Substitute the value of x=1 in above equation
5×1+2y=7
2y=7-5
2y=2
y=1

---

5. If x+y=4 and x-y=10, then value of x and y is

(a) 7, -3

(b) -3,7

(c) 7,3

(d) 3,7

Answer

Answer: (a) 7, -3

Explanation: x+y=4 ……………………………………………(1)
x-y=10……………………………………………..(2)
Add equation (1) and (2)
2x = 14
x = 7
Substitute value of x in equation (1)
7+y=4
y = -3

---

 

6. If 3x+4y=12 and 3x+4y =17, then lines are

(a) parallel

(b) intersecting

(c)coincident

(d) All of the above

Answer

Answer: (a) parallel

Explanation:

a₁ = 3, b₁=4 and c₁=-12

a₂=3, b₂-=4 and c₂=-17

On comparing ratios, we get

a₁ /a₂ = b₁ /b₂ ≠ c₁ /c₂

So, the lines are parallel.

---

 

Pair of Linear Equations in Two Variables Class 10 MCQ with Answers

7. The sum of two numbers is 50 and one number is four times the other. The numbers are

(a) 35,15

(b) 40,20

(c) 25,25

(d) 10,40

Answer

Answer: (d) 10,40

Explanation: Let the first number be x.
And the second number be 4x.
According to question,
x+4x=50
5x=50
x=10
So, the first number is 10 and second number is 40

---

8. The pair of equations 9m+10n+11=0 and 18m+20n+33=0 have

(a) a unique solution

(b)exactly two solutions

(c) no solution

(d)infinitely many solutions

Answer

Answer: (c) no solution

Explanation: Given equations are 9m+10n+11=0 and 18m+20n+33=0

Comparing with standard form

a₁ = 9, b₁=10 and c₁=11

a₂=18, b₂-=20 and c₂=33

a₁/a₂ = 9/18 = 1/2 , b₁/b₂ = 10/20 = 1/2, c₁/c₂ = 11/33 = 1/3

a₁ /a₂ = b₁ /b₂ ≠ c₁ /c₂

So, the pair of equations has no solution.

---

 

9. If the pair of linear equations has no solution, then the lines representing these equations will

(a)coincide

(b)intersect at one point

(c)parallel to each other

(d) parallel to y-axis

Answer

Answer: (c)parallel to each other

Explanation: If the pair of linear equations has no solution, then the lines representing these equations will be parallel to each other.

---

 

10. A pair of linear equations which has a unique solution x=1 and y=-2 is

(a) x+y =1; 2x+3y=-6

(b) x+2y=9; 3x+7y=0

(c) 2x+2y=-2; 7x-8y=23

(d) x+4y=0; 4y+3x=1

Answer

Answer: (c) 2x+2y=-2; 7x-8y=23

Explanation: If x=1 and y=-2 is unique solution of any pair of equations, then these values must satisfy that pair of equations.
Now, 2x+2y = -2
LHS = 2x+2y = 2(1) +2(-2) =2-4 = -2 = RHS
LHS = 7x-8y = 7(1)-8(-2) = 7+16 = 23 = RHS

---

 

11.  If the lines 3x+4(k+1)y+4=0 and 2x+8y+12=0 are parallel then, value of k is

(a)1

(b)2

(c)3

(d)4

Answer

Answer: (b)2

Explanation: The condition for parallel lines is

a₁ /a₂ = b₁ /b₂ ≠ c₁ /c₂

3/2 = (4(k+1))/8
3=(k+1)
K=2

---

 

12.  If 3x+7y=10 and 6y-3x=16, then what will be the value of ‘m’ in y = mx+3

(a)4/3

(b)-4/3

(c)3/4

(d) -3/4

Answer

Answer: (c)3/4

Explanation: Given equations are 3x+7y=10…………..(1)

and 6y-3x=16………………..(2)

Add equation (1) and (2)

3x+7y+ 6y-3x=16+10

13y=26

y = 2

Substitute value of y in equation (1)

3x+7(2) = 10

3x=-4

x = -4/3

Now, y = mx+3…………………………(3)

Substitute values of x and y in equation (3)

2 = m (-4/3) +3

2= (-4m+9)/3

-4m+9 = 6

-4m=-3

m = 3/4

---

13. Graphically, the pair of equations 2x+4y=12 and x+2y=8 represents two lines which are

(a)intersecting at one point

(b)parallel

(c)intersecting at two points

(d)coincident

Answer

Answer: (b)parallel

Explanation: 2x+4y=12

X	0	6
Y	3	0

x+2y=8

X	8	0
Y	0	4

---

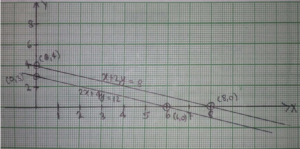
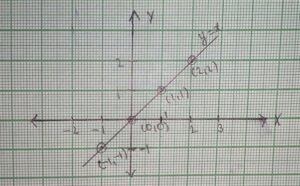
14. The graph of y=x is a line

(a)Parallel to x-axis

(b)parallel to y axis

(c) Passing through the origin

(d) perpendicular to x-axis

Answer

Answer: (c) Passing through the origin

Explanation: y=x

X	0	1	2	-1
Y	0	1	2	-1

---

 

15. Rohan bought some mobile and watch for his shop. The number of watches are 2 more than 6 times of mobile also number of watches are 5 more than 3 times pf mobile. Then number of mobile and watches will be

(a)(1,8)

(b)(8,1)

(c) (1,1)

(d) (8,8)

Answer

Answer: (a)(1,8)

Explanation: Let number of mobiles be x and number of watches be y.
According to question
y = 6x + 2
y = 3x + 5

X	0	-1/3
y = 6x +2	2	0

X	0	-5/3
y = 3x+5	5	0

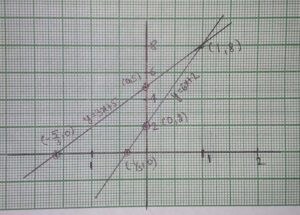
From the above graph we can observe that both lines are intersecting at point (1,8).

So, number of mobiles is 1 and number of watches is 8

---

 

 

Pair of Linear Equations in Two Variables Class 10 MCQ with Answers

16. Check the equation x+5y=10 and 0.4x-2y =4 is consistent or inconsistent

(a)Consistent

(b)Inconsistent

Answer

Answer: (a)Consistent

Explanation: x+5y=10

X	0	10
y = (10-x)/5	2	0

0.4x-2y =4 or 4x-20y =40 or x-5y = 10

X	0	10
y = (x-10)/5	-2	0

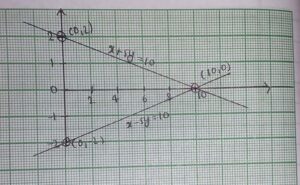
So, from the above graph we can observe that there is point which is common to both lines. So, solution of the pair of equations is x = 10 and y = 0 i.e., the pair of linear equations is consistent.

---

 

17. For what value of k, do the equations 7x+14y=21 and 14x-2ky=42 represent coincident lines

(a)-14

(b)14

(c) 11

(d)7

Answer

Answer: (a)-14

Explanation: For coincident lines,

a₁ /a₂ = b₁ /b₂ = c₁ /c₂

7/14=14/(-2k)=21/42

1/2=7/(-k)

k = -14

---

 

18.  If in the equation 0.75x+0.25y=9, the value of x is 1, then what will be the value of y

(a)25

(b)33

(c)11

(d)25.05

Answer

Answer: (b)33

Explanation: 0.75x+0.25y=9

Substitute x=1

0.75(1)+0.25y=9

0.25y=9-0.75

0.25y = 8.25

y = 33

---

 

19. Solution of equations  2/x – 5/y = 10 and  1/x + 3/y = -5 is

(a) (5/11 , -20/11 )

(b) (11/5 ,-11/20 )

(c) (-20/11, 5/11 )

(d) ( 5/11, 1/11)

Answer

Answer: (b) (11/5 ,-11/20 )

Explanation: 2(1/x) –5( 1/y) = 10 and 1(1/x) +3 ( 1/y) = -5

Let 1/x = p and 1/y = q

Then, 2p-5q = 10………. (1)

and p + 3q =-5

p = -5-3q

Put value of p in equation (1)

2(-5-3q) – 5q =10

-10-6q – 5q =10

-11q = 20

q = -20/11

p = -5 -3(-20/11)= -5 + 60/11

p = (-55+60)/11 = 5/11

Now, p = 1/x= 5/11

x = 11/5

and q = 1/y=(-20)/11

y = (-11)/20

---

 

20. Which of the following is not a solution of 3x+y=9

(a) (2,3)

(b) (1.2,5.4)

(c) (3,3)

(d) (1,6)

Answer

Answer: (c) (3,3)

Explanation: For (3,3)

LHS = 3(3)+3 = 9+3 = 12 ≠ RHS

---

21. Find the value of p for which the system of equations px+7y=20 and x-y=2 will represent intersecting lines

(a)p ≠ -7

(b)p=-7

(c)p=7

(d) p

Answer

Answer: (a)p ≠ -7

Explanation: The condition for intersecting lines is
a₁ /a₂  ≠ b₁ /b₂
p/1 ≠7/(-1)
P ≠-7

---

 

Pair of Linear Equations in Two Variables Class 10 MCQ with Answers

22. Find the solution of the following pair of linear equation: 2x- √3 y = 6 and x-y=8

(a) ((-6+√3)/2, (10+√3)/2 )

(b)  ((-2+√3)/2,  (-6+√3)/2)

(c)  ((2+√3)/2, (-2+√3)/2 )

(d)  ((6+√3)/2, (-10+√3)/2 )

Answer

Answer: (d)  ((6+√3)/2, (-10+√3)/2 )

Explanation: For given equations 2x-√3 = 6 and x-y=8

x = (6+√3 )/2

Substitute x = (6+√3 )/2

(6+√3 )/2-y=8

6+√3 – 2 y = 16

6+√3-16 = 2y

y = (-10+√3 )/2

---

 

23.  The cost of 2 dolls and 5 cars is Rs9 and the cost of 4 dolls and 8 cars is Rs 16. Find the cost of each doll and each car.

(a)(1,3)

(b)(2,1)

(c)(2,4)

(d)(4,3)

Answer

Answer: (b)(2,1)

Explanation: Let cost of a doll is Rs x and cost of a car is Rs y
According to question
2x+5y=9……………….(1)
4x+8y=16……………….(2)
x + 2y = 4
x = 4-2y…………….(3)
Substitute x in equation (1)
2(4-2y) + 5y = 9
8-4y+5y = 9
y = 1
substitute value of y in equation (3)
x = 4-2(1)
x=2
Cost of a doll is Rs 2 and cost of a car is Rs1.

---

 

24. Find the value of p, if 2x+py=7 and px+12y=21 are coincident lines

(a)p=8√2

(b)p=2√3

(c)p=3

(d)p=2√6

Answer

Answer: (d)p=2√6

Explanation: The condition for coincident lines is
a₁ /a₂ = b₁ /b₂ = c₁ /c₂
2/p=p/12
P² =24
p = 2√6

---

 

25. Solution of equations 2/(x-10) – 1/(y-2) = 10 and 1/(x-10) +2/(y-2)  = 15 is

(a) (71/7, 9/4 )

(b) (7/71 , 4/9 )

(c) (7,4)

(d) (4,7)

Answer

Answer: (a) (71/7, 9/4 )

Explanation: 2(1/(x-10)) – 1/(y-2) = 10 and 1/(x-10) + 2(1/(y-2)) = 15

Let (1/(x-10)) = p and (1/(y-2)) = q

Now, 2p -q = 10

q = 2p – 10

p + 2q = 15

Substituting value of q

p + 2(2p – 10) = 15

p + 4p -20 =15

5p = 35 and p = 7

q = 2(7) – 10 = 4

Now, p = (1/(x-10)) = 7

1 = 7x -70

7x = 71 and x =71/7

q = (1/(y-2)) = 4

1 = 4y – 8

4y = 9 and y = 9/4

---

 

MCQ Questions for Class 10 Maths

• Real Numbers Class 10 MCQ with Answers
• Polynomials Class 10 MCQ with Answers
• Pair of Linear Equations in Two Variables
• MCQ Questions on Quadratic Equations for Class 10
• Arithmetic Progressions
• Triangles Class 10 MCQ with Answers
• Coordinate Geometry
• Introduction to Trignometry
• Some Applications of Trigonometry Class 10 MCQ with Answers
• Circles Class 10 MCQ with Answers
• Constructions Class 10 MCQ with Answers
• Areas Related to Circles
• Surface Areas and Volumes
• Statistics Class 10 MCQ with Answers
• Probability Class 10 MCQ