Conservation of Momentum

Newton’s second law of motion focuses on rate of change of momentum. We know that momentum is mass multiplied by velocity. Law of conservation of momentum is also an important concept of physics and it is useful in understanding physical phenomena.

According to law of conservation of momentum:

Momentum is neither created nor destroyed.

In other words, when two or more bodies act upon one another, their total momentum remains constant or conserved provided no external forces are acting. It states that whenever one body gains momentum, the other body loses an equal amount of momentum.

We can understand this concept with the help of some examples.

Suppose two bodies, a truck and a car, are moving in the same direction towards east but with different speeds or velocities. Let the mass of truck be m₁ and its velocity be u₁ so that its initial momentum is m₁u₁. Let the mass of car be m₂ and its velocity be u₂ so that the initial momentum of the car is m₂u₂ Thus, the total momentum of the truck and car before collision is m₁u_{1 +} m₂u_2.

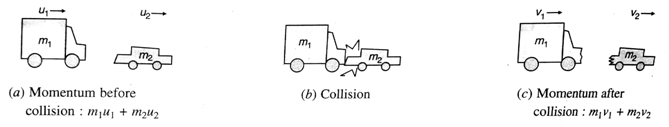
Suppose the truck and car collide for a short time t and due to collision, the velocities of the truck and the car will change. Let the velocity of the truck after the collision be v₁, and the velocity of the car after the collision be v₂. So, the momentum of the truck after collision will be m₁v₁, and the momentum of the car after the collision will be m₂v₂. So, the total momentum of truck and car after collision will be m₁v₁+ m₂v₂

Suppose that during collision, the truck exerts a force F₁ on the car and, in turn, the car exerts a force F₂ on the truck.

When force F₁ acts on the car for time t, the velocity of the car changes from u₂ to v₂. So, acceleration of car (a₂)

a₂ = (v₂  – u₂)/t

But force = mass × acceleration

F₁ = m₂  × (v₂  – u₂)/t

When force F₂ acts on the truck for time t, the velocity of the truck changes from u₁ to v₁. So, acceleration of truck (a₁)

a₁ = (v₁  – u₁)/t

But force = mass × acceleration

F₂ = m₁  × (v₁  – u₁)/t

Now, the force F₁ exerted by truck is ‘action’ and the force F₂ exerted by the car is the ‘reaction’. But according to the third law of motion, the action and reaction are equal and opposite. That is,

F₁ = -F₂

Substituting values from above

m₂  × (v₂  – u₂)/t = – m₁  × (v₁  – u₁)/t

Cancelling (t) from both sides we get,

m₂  × (v₂  – u₂) = – m₁  × (v₁  – u₁)

m₂v₂  – m₂u₂ = -m₁v₁  + m₁u₁

m₂v₂  + m₁v₁ = m₂u₂ + m₁u₁

m₁u₁  + m₂u₂ = m₁v₁ + m₂v₂

Now, m₁u₁  + m₂u₂ is total momentum of truck and car before collision whereas m₁v₁ + m₂v₂ is total momentum of car and truck after collision.

Therefore, Total momentum before collision = Total momentum after collision

This is law of conservation of momentum.

Applications of law of conservation of momentum –

1. The chemicals inside a rocket burn and produce high velocity blast of hot gases. These gases pass out through the tail nozzle of the rocket in the downwards direction with tremendous speed and the rocket moves up to balance the momentum of the gases.
2. In jet aeroplane, a large volume of gases produced by the combustion of fuel is allowed to pass through a jet in the backward direction. Due to the very high speed, the backward rushing gases have a large momentum. To balance this momentum, jet aeroplane moves forward with great speed.
3. When a bullet is fired from the gun, the bullet has a momentum. The bullet imparts an equal and opposite momentum to the gun due to which the gun recoils and jerks backwards.

Questions related to the Law of Conservation of Momentum from NCERT textbook – Page 126 and 127

Question 3:-

From a rifle of mass 4 kg, a bullet of mass 50 g is fired with an initial velocity of 35 ms^-1. Calculate the initial recoil velocity of the rifle.

Solution –

Momentum of bullet = mass of bullet × velocity of bullet

= (50)/(1000) Kg  × 35 ms^-1

= 0.05 Kg x 35 ms^-1

= 1.75 Kg ms^-1

Momentum of rifle = mass of rifle × velocity of rifle

= 4 kg × v ms^-1

= 4 v kg ms^-1

According to law of conservation of momentum,

Momentum of bullet = Momentum of rifle

1.75 = 4v

v = 1.75/4

v = 0.4375 ms^-1

Question 4:-

Two objects of masses 100 g and 200 g are moving along the same line and direction with velocities of 2 ms^-1 and 1 ms^-1, respectively. They collide and after the collision, the first object moves at a velocity of 1.67 ms^-1. Determine the velocity of the second object.

Solution –

Momentum of first object before collision = mass of object × velocity of object

= 100/1000 Kg × 2 ms^-1

= 0.1 kg × 2 ms^-1

= 0.2 kg ms^-1

Momentum of second object before collision = mass of object × velocity of object

= 200/1000 Kg × 1 ms^-1

= 0.2 kg × 1 ms^-1

= 0.2 kg ms^-1

Total momentum before collision = 0.2 kg ms^-1 + 0.2 kg ms^-1

= 0.4 kg ms^-1

Momentum of first object after collision = 100/1000 Kg × 1.67 ms^-1

= 0.1 Kg × 1.67 ms^-1

= 0.167 kg ms^-1

Momentum of second object after collision = 200/1000 Kg × v ms^-1

= 0.2 v kg ms^-1

Total momentum after collision = 0.167 kg ms^-1 + 0.2 v kg ms^-1

Total momentum after collision = total momentum before collision

0.4 = 0.167 + 0.2v

0.2v = 0.233

v = 0.233/0.2

v = 1.165 ms^-1

NCERT textbook – Page 128 and 129

Question 11:-

Two objects, each of mass 1.5 kg, are moving in the same straight line but in opposite directions. The velocity of each object is 2.5 ms^-1 before the collision during which they stick together. What will be the velocity of the combined object after collision?

Solution –

Mass of first object m₁ = 1.5 kg

Velocity of first object v₁ = 2.5 ms^-1

Momentum of first object = m₁ × v₁

= 1.5 Kg × 2.5 ms^-1

= 3.75 Kg ms^-1

Mass of second object m₂ = 1.5 kg

Velocity of first object v₂ = – 2.5 ms^-1

Momentum of second object = m₂ × v₂

= 1.5 kg × 2.5 ms^-1

= -3.75 Kg ms^-1

Total momentum before collision= 3. 75 – 3.75 = 0 kg ms^-1

Total mass of combined objects = m₁+m₂ = 1.5 kg+1.5 kg = 3.0 kg

Let velocity of combined objects be v

Total momentum after collision = (m₁+m₂) v

= 3v kg ms^-1

0 = 3v

v=0 m/s 

Question 13:-

A hockey ball of mass 200 g travelling at 10 ms^-1 is struck bya hockey stick so as to return italong its original path with a velocity at 5 ms^-1. Calculate the change of momentum occurredin the motion of the hockey ball by the force applied by the hockey stick.

Solution –

Mass of hockey ball m₁ = 200 g

= 200/1000 kg

= 0.2 kg

Initial velocity v₁ = 10 m/s

Initial momentum = 0.2×10 kg m/s = 2 kg m/s

Mass of hockey ball = 0.2 kg

Final velocity v₂ = -5 m/s

Final momentum = m₂ × v₂ = -1 kg m/s

Change in momentum = final momentum – initial momentum

= -1 – 2 = -3 kg m/s

Question 15:-

An object of mass 1 kg travelling in a straight line with a velocity of 10 ms^-1collides with, and sticks to, a stationary wooden block of mass 5 kg. Then they both move off together in the same straight line. Calculate the total momentum just before the impact and just after the impact. Also, calculate the velocity of the combined object.

Solution –

Mass of object m₁ = 1 kg

Velocity of object v₁ =10 m/s

Momentum of object = m₁ × v₁

= 1×10 kg m/s

= 10 kg m/s

Mass of wooden block m₂ = 5 kg

Velocity of wooden block v₂ = 0

Momentum of wooden block = m₂ × v₂

= 5 × 0 = 0 kg m/s

Total momentum before impact = 10+0 = 10 kg m/s

According to law of conservation of momentum, the momentum before and after the impact will be same

Total momentum = 10 kg m/s

Total mass of object and wooden block = 6 kg

Velocity of wooden block and object = v m/s

10 = 6×v

v=1.67 m/s

The law of conservation of momentum states that the momentum of a system is neither created nor destroyed. Whenever two bodies interact with each other, one body loses momentum and other body gains an equal amount of momentum. This law explains why a gun recoils when a bullet is shot from it. It also explains the movement of rocket and jet aeroplanes. This law is useful in understanding the situation when two bodies collide.