NCERT Solutions For Class 10 Maths Chapter 7 Exercise 7.1 Coordinate Geometry

NCERT Solutions for Class 10 Maths Chapter 7 Exercise 7.2

Download NCERT Solutions For Class 10 Maths Chapter 7 Exercise 7.1 Coordinate Geometry. Ex 7.1 class 10 contains 10 questions, for which detailed answers have been provided in this note. In case you are looking at studying the remaining Exercise for class 10 maths chapter 7 NCERT solutions, you can click the link at the end of this Note.

Category NCERT Solutions for Class 10
Subject Maths
Chapter Chapter 7
Exercise Exercise 7.1
Chapter Name Coordinate Geometry

NCERT Solutions For Class 10 Maths Chapter 7 Exercise 7.1 Coordinate Geometry

1. Find the distance between the following pair of point

i. (2, 3), (4, 1)

Using distance formula
Distance = \sqrt { (x_{ 1 }-x_{ 2 })^{ 2 }+(y_{ 1 }-y_{ 2 })^{ 2 } }
= \sqrt { (2-4)^{ 2 }+(3-1)^{ 2 } }
= \sqrt { (-2)^{ 2 }+(2)^{ 2 } }
= \sqrt { 4+4 }
= \sqrt { 8 }
= 2\sqrt { 2 }

ii. (-5, 7), (-1, 3)

Using distance formula
Distance = \sqrt { (x_{ 1 }-x_{ 2 })^{ 2 }+(y_{ 1 }-y_{ 2 })^{ 2 } }
= \sqrt { (-5-(-1))^{ 2 }+(7-3)^{ 2 } }
= \sqrt { (-4)^{ 2 }+(4)^{ 2 } }
= \sqrt { 16+16 }
= \sqrt { 32 }
= 4\sqrt { 2 }

iii. (a, b), (-a, -b)

Using distance formula
Distance = \sqrt { (x_{ 1 }-x_{ 2 })^{ 2 }+(y_{ 1 }-y_{ 2 })^{ 2 } }
= \sqrt { (a-(-a))^{ 2 }+(b-(-b))^{ 2 } }
= \sqrt { (2a)^{ 2 }+(2b)^{ 2 } }
= \sqrt { 4(a^{ 2 }+b^{ 2 }) }
= 2\sqrt { a^{ 2 }+b^{ 2 } }

2. Find the distance between the two points (0, 0) and (36, 15).

Using distance formula
Distance = \sqrt { (x_{ 1 }-x_{ 2 })^{ 2 }+(y_{ 1 }-y_{ 2 })^{ 2 } }
= \sqrt { (36-0)^{ 2 }+(15-0)^{ 2 } }
= \sqrt { 36^{ 2 }+15^{ 2 } }
= \sqrt { 1296+225 }
= \sqrt { 1521 }
= 39

3. Determine if the points (1, 5), (2, 3) and (-2, -11) are collinear.

Let A, B and C denote the points (1, 5), (2, 3) (-2, -11) respectively
Using distance formula
AB = \sqrt { (1-2)^{ 2 }+(5-3)^{ 2 } } = \sqrt { (-1)^{ 2 }+(2)^{ 2 } } = \sqrt { 1+4 } = \sqrt { 5 }
BC = \sqrt { (2-(-2))^{ 2 }+(3-(-11))^{ 2 } } = \sqrt { 4^{ 2 }+14^{ 2 } } = \sqrt { 16+196 } = \sqrt { 212 }
CA = \sqrt { (-2-1)^{ 2 }+(-11-5)^{ 2 } } = \sqrt { (-3)^{ 2 }+(-16)^{ 2 } } = \sqrt { 9+256 }
∵ AB + BC ≠ CA
BC + CA ≠ BA
CA + AB ≠ CB
∴ A, B and C are non-collinear

Class 10 ex 7.1 chapter 7 Maths NCERT Solutions

4. Check whether (5, -2), (6, 4) and (7, -2) are the vertices of an isosceles triangle.

Let A, B and C denote the points (5, -2), (6, 4) (7, -2) respectively
Using distance formula
AB = \sqrt { (5-6)^{ 2 }+(-2-4)^{ 2 } } = \sqrt { (-1)^{ 2 }+(-6)^{ 2 } } = \sqrt { 1+36 } = \sqrt { 37 }
BC = \sqrt { (6-7)^{ 2 }+(4-(-2) } = \sqrt { (-1)^{ 2 }+6^{ 2 } } = \sqrt { 1+36 } = \sqrt { 37 }
CA = \sqrt { (7-5)^{ 2 }+(-2-(-2))^{ 2 } } = \sqrt { (2)^{ 2 }+(0)^{ 2 } } = \sqrt { 2^{ 2 } } = 2
∵ AB = BC
∴ Two sides of triangle are equal in length. Therefore, ABC is an isosceles triangle

5. In a classroom, 4 friends are seated at the points A, B, C and D as shown. Champa and Chameli walk into the class and after observing for a few minutes Champa asks Chameli, “Don’t you think ABCD is a square?” Chameli disagrees. Using distance formula, find which of them is correct.

Points A, B, C and D are respectively (3, 4), (6, 7), (9, 4) and (6, 1)

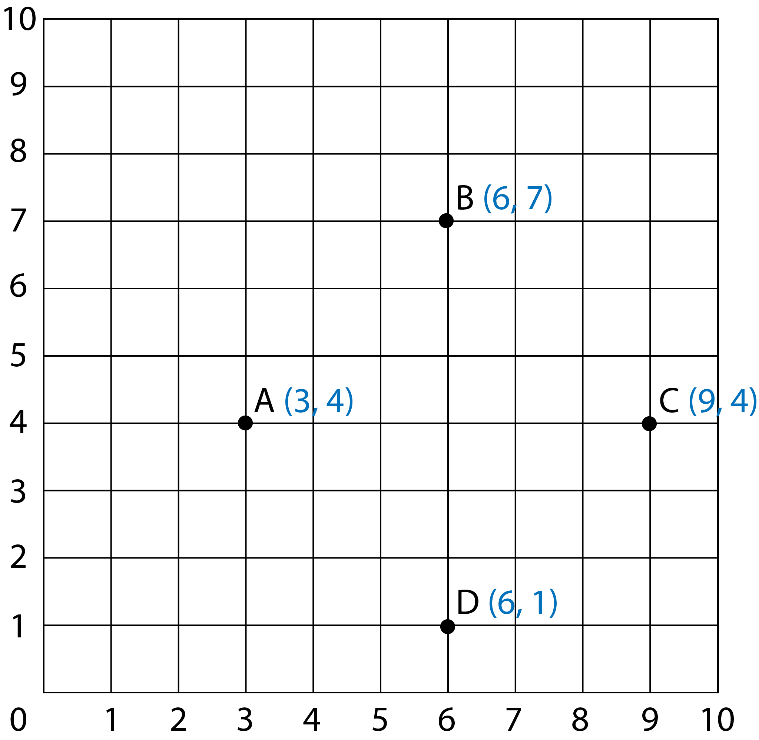
NCERT Solutions For Class 10 Maths Chapter 7 Exercise 7.1 Question 5 Coordinate Geometry

Using distance formula
Length of sides
AB = \sqrt { (3-6)^{ 2 }+(4-7)^{ 2 } } = \sqrt { (-3)^{ 2 }+(-3)^{ 2 } } = \sqrt { 2\times 3^{ 2 } } = 3\sqrt { 2 }
BC = \sqrt { (6-9)^{ 2 }+(7-4)^{ 2 } } = \sqrt { (-3)^{ 2 }+3^{ 2 } } = \sqrt { 2\times 3^{ 2 } } = 3\sqrt { 2 }
CD = \sqrt { (9-6)^{ 2 }+(4-1)^{ 2 } } = \sqrt { 3^{ 2 }+3^{ 2 } } = \sqrt { 2\times 3^{ 2 } } = 3\sqrt { 2 }
DA = \sqrt { (6-3)^{ 2 }+(1-4)^{ 2 } } = \sqrt { 3^{ 2 }+(-3)^{ 2 } } = \sqrt { 2\times 3^{ 2 } } = 3\sqrt { 2 }
∵ AB = BC = CD = DA
⇒ ABCD is a rhombus
Length of diagonals
AC = \sqrt { (3-9)^{ 2 }+(4-4)^{ 2 } }  = \sqrt { (-6)^{ 2 }+0^{ 2 } }  = \sqrt { 6^{ 2 } }  = 6
BD = \sqrt { (6-6)^{ 2 }+(7-1)^{ 2 } }  = \sqrt { 0^{ 2 }+6^{ 2 } } = \sqrt { 6^{ 2 } }  = 6
∵ AC = BD
∴ Diagonals are equal
A rhombus having diagonals of equal length is a square
⇒ ABCD is a square
Hence, Champa is correct




Coordinate Geometry Class 10 NCERT Solutions

6. Name the type of quadrilateral formed, if any, by the following points, and give reasons for your answer:

i. (-1, -2), (1, 0), (-1, 2), (-3, 0)

Let A, B, C and D denote the points (–1, –2), (1, 0), (–1, 2) and (–3, 0) respectively
Using distance formula
Length of sides
AB = \sqrt { (-1-1)^{ 2 }+(-2-0)^{ 2 } } = \sqrt { (-2)^{ 2 }+(-2)^{ 2 } } = \sqrt { 2\times 2^{ 2 } } = 2\sqrt { 2 }
BC = \sqrt { (1-(-1))^{ 2 }+(0-2)^{ 2 } }  = \sqrt { 2^{ 2 }+(-2)^{ 2 } } = \sqrt { 2\times 2^{ 2 } } = 2\sqrt { 2 }
CD = \sqrt { (-1-(-3))^{ 2 }+(2-0)^{ 2 } }  = \sqrt { 2^{ 2 }+2^{ 2 } } = \sqrt { 2\times 2^{ 2 } } = 2\sqrt { 2 }
DA = \sqrt { (-3-(-1))^{ 2 }+(0-(-2))^{ 2 } } = \sqrt { (-2)^{ 2 }+2^{ 2 } } = \sqrt { 2\times 2^{ 2 } } = 2\sqrt { 2 }
Length of diagonals
AC = \sqrt { (-1-(-1))^{ 2 }+(-2-2)^{ 2 } }  = \sqrt { (0^{ 2 }+(-4)^{ 2 } }  = \sqrt { 4^{ 2 } } = 4
BD = \sqrt { (1-(-3))^{ 2 }+(0-0)^{ 2 } }  = \sqrt { 4^{ 2 }+0^{ 2 } } = \sqrt { 4^{ 2 } } = 4
∵ All sides are equal (AB = BC = CD = DA) and both the diagonals are of same length (AC = BD)
∴ The quadrilateral formed by these points is a square

ii. (-3, 5), (3, 1), (0, 3), (-1, -4)

Let A, B, C and D denote the points (-3, 5), (3, 1), (0, 3) and (-1, -4) respectively
Using distance formula
Length of sides
AB = \sqrt { (-3-3)^{ 2 }+(5-1)^{ 2 } }  = \sqrt { (-6)^{ 2 }+4^{ 2 } }  = \sqrt { 36+16 } = \sqrt { 52 } = 2\sqrt { 13 }
BC = \sqrt { (3-0)^{ 2 }+(1-3)^{ 2 } }  = \sqrt { 3^{ 2 }+(-2)^{ 2 } }  = \sqrt { 9+4 } = \sqrt { 13 }
CD = \sqrt { (0-(-1))^{ 2 }+(3-(-4))^{ 2 } }  = \sqrt { 1^{ 2 }+7^{ 2 } }  = \sqrt { 1+49 } = \sqrt { 50 } = 5\sqrt { 2 }
DA = \sqrt { (-1-(-3))^{ 2 }+(-4-5)^{ 2 } }  = \sqrt { 2^{ 2 }+9^{ 2 } }  = \sqrt { 4+81 } = \sqrt { 85 }
Length of diagonals
AC = \sqrt { (-3-0)^{ 2 }+(5-3)^{ 2 } }  = \sqrt { (-3)^{ 2 }+2^{ 2 } }  = \sqrt { 9+4 } = \sqrt { 13 }
BD = \sqrt { (3-(-1))^{ 2 }+(1-(-4))^{ 2 } }  = \sqrt { 4^{ 2 }+5^{ 2 } }  = \sqrt { 16+25 } = \sqrt { 41 }
∵ AC + CB = \sqrt { 13 } + \sqrt { 13 } = 2\sqrt { 13 } = AB
∴ ABC lie on the same line
⇒ ABCD is not a quadrilateral

iii. (4, 5), (7, 6), (4, 3), (1, 2)

Let A, B, C and D denote the points (4, 5), (7, 6), (4, 3) and (1, 2) respectively
Using distance formula
Length of sides
AB = \sqrt { (4-7)^{ 2 }+(5-6)^{ 2 } }  = \sqrt { (-3)^{ 2 }+(-1)^{ 2 } }  = \sqrt { 9+1 } = \sqrt { 10 }
BC = \sqrt { (7-4)^{ 2 }+(6-3)^{ 2 } }  = \sqrt { 3^{ 2 }+3^{ 2 } }  = \sqrt { 2\times 3^{ 2 } } = 3\sqrt { 2 }
CD = \sqrt { (4-1)^{ 2 }+(3-2)^{ 2 } }  = \sqrt { 3^{ 2 }+1^{ 2 } }  = \sqrt { 9+1 } = \sqrt { 10 }
DA = \sqrt { (1-4)^{ 2 }+(2-5)^{ 2 } }  = \sqrt { (-3)^{ 2 }+(-3)^{ 2 } }  = \sqrt { 2\times 3^{ 2 } }   = 3\sqrt { 2 }
Length of diagonals
AC = \sqrt { (4-4)^{ 2 }+(5-3)^{ 2 } }  = \sqrt { 0^{ 2 }+2^{ 2 } }  = \sqrt { 2^{ 2 } } = 2
BD = \sqrt { (7-1)^{ 2 }+(6-2)^{ 2 } }  = \sqrt { 6^{ 2 }+4^{ 2 } } = \sqrt { 36+16 } = \sqrt { 52 } = 2\sqrt { 13 }
∵ Opposite sides are equal (AB = CD and BC = DA) and the diagonals are of unequal lengths
∴ ABCD is a parallelogram

7. Find the point on the x-axis which is equidistant form (2, -5) and (-2, 9).

Let A and B denote the points (2, -5) and (-2, 9) respectively
Let the point C(x, 0) on x-axis be equidistance from A and B
∴ AC = BC
\sqrt { (2-x)^{ 2 }+(-5-0)^{ 2 } } = \sqrt { (-2-x)^{ 2 }+(9-0)^{ 2 } }
\sqrt { 2^{ 2 }+x^{ 2 }-4x+25 } = \sqrt { 2^{ 2 }+x^{ 2 }+4x+81 }
On squaring both sides
22 + x2 – 4x + 25 = 22 + x2 + 4x + 81
-4x + 25 = 4x + 81
8x = -81 + 25
8x = -56
x = -7
Therefore, the point (-7, 0) is the point on x-axis that is equidistant from A and B




Coordinate Geometry Class 10 Ex 7.1 NCERT Solutions

8. Find the values of y for which the distance between the points P(2, -3) and Q(10, y) is 10 units.

According to the question
PQ = 10
\sqrt { (2-10)^{ 2 }+(-3-y)^{ 2 } }   = 10
\sqrt { (-8)^{ 2 }+3^{ 2 }+y^{ 2 }+6y } = 10
\sqrt { y^{ 2 }+6y+73 } = 10
On squaring both sides
y2 + 6y + 73 = 100
y2 + 6y – 27 = 0
By Splitting the middle term
y2 + 9y – 3y – 27 = 0
y(y + 9) – 3(y + 9) = 0
(y – 3)(y + 9) = 0
⇒ y = 3, -9
Therefore, for y = 3 and y = -9, the distance between the points P and Q is 10 units.

9. If Q(0, 1) is equidistant from P(5, -3) and R(x, 6), find the values of x. Also find the distances QR and PR.

According to the question
PQ = QR
\sqrt { (0-5)^{ 2 }+(1-(-3))^{ 2 } } =\quad \sqrt { (0-x)^{ 2 }+(1-6)^{ 2 } }
\sqrt { 5^{ 2 }+4^{ 2 } } =\quad \sqrt { x^{ 2 }+(-5)^{ 2 } }
\sqrt { 25+16 } =\quad \sqrt { x^{ 2 }+25 }
On squaring both sides
25 + 16 = x2 + 25
x2 = 16
⇒ x = ±4
If x = +4
PR = \sqrt { (5-4)^{ 2 }+(-3-6)^{ 2 } }  = \sqrt { 1^{ 2 }+(-9)^{ 2 } }  = \sqrt { 1+81 } = \sqrt { 82 }
QR = \sqrt { (0-4)^{ 2 }+(1-6)^{ 2 } }  = \sqrt { (-4)^{ 2 }+(-5)^{ 2 } }  = \sqrt { 16+25 } = \sqrt { 41 }
If x = -4
PR = \sqrt { (5-(-4))^{ 2 }+(-3-6)^{ 2 } } = \sqrt { 9^{ 2 }+(-9)^{ 2 } } = \sqrt { 2\times 9^{ 2 } } = 9\sqrt { 2 }
QR = \sqrt { (0-(-4))^{ 2 }+(1-6)^{ 2 } }  = \sqrt { 4^{ 2 }+(-5)^{ 2 } }  = \sqrt { 16+25 } = \sqrt { 41 }

10. Find a relation between x and y such that the point (x, y) is equidistant form the point (3, 6) and (-3, 4).

Let A, B and C denote the points (x, y), (3, 6) and (-3, 4) respectively
According to the question
AB = AC
\sqrt { (x-3)^{ 2 }+(y-6)^{ 2 } } =\sqrt { (x-(-3))^{ 2 }+(y-4)^{ 2 } }
\sqrt { x^{ 2 }+3^{ 2 }-6x+y^{ 2 }+6^{ 2 }-12y } =\sqrt { x^{ 2 }+3^{ 2 }+6x+y^{ 2 }+4^{ 2 }-8y }
On squaring both sides
x2 + 9 – 6x + y2 + 36 – 12y = x2 + 9 + 6x + y2 + 16 – 8y
12x + 4y – 20 = 0
3x + y – 5 = 0

NCERT Solutions for ex 7.1 class 10 Maths Chapter 7 Coordinate Geometry, has been designed by the NCERT to test the knowledge of the student on the topic Distance Formula

Download NCERT Solutions For class 10 ex 7.1 Maths chapter 7 Coordinate Geometry

NCERT Solutions for Class 10

CBSE Notes for Class 10

MCQ Questions for Class 10

Leave a Comment