Arinjay Academy » Maths Class 10 » NCERT Solutions For Class 10 Maths Chapter 3 Exercise 3.6 – Pair of Linear Equations in two Variables

NCERT Solutions For Class 10 Maths Chapter 3 Exercise 3.6 – Pair of Linear Equations in two Variables

Download NCERT Solutions For Class 10 Maths Chapter 3 Exercise 3.6 – Pair of Linear Equations in two Variables. This Exercise contains 2 questions, for which detailed answers have been provided in this note. In case you are looking at studying the remaining Exercise for Class 10 for Maths NCERT solutions for other Chapters, you can click the link at the end of this Note.

NCERT Solutions For Class 10 Maths Chapter 3 Exercise 3.6 – Pair of Linear Equations in two Variables

NCERT Solutions For Class 10 Maths Chapter 3 Exercise 3.6 – Pair of Linear Equations in two Variables

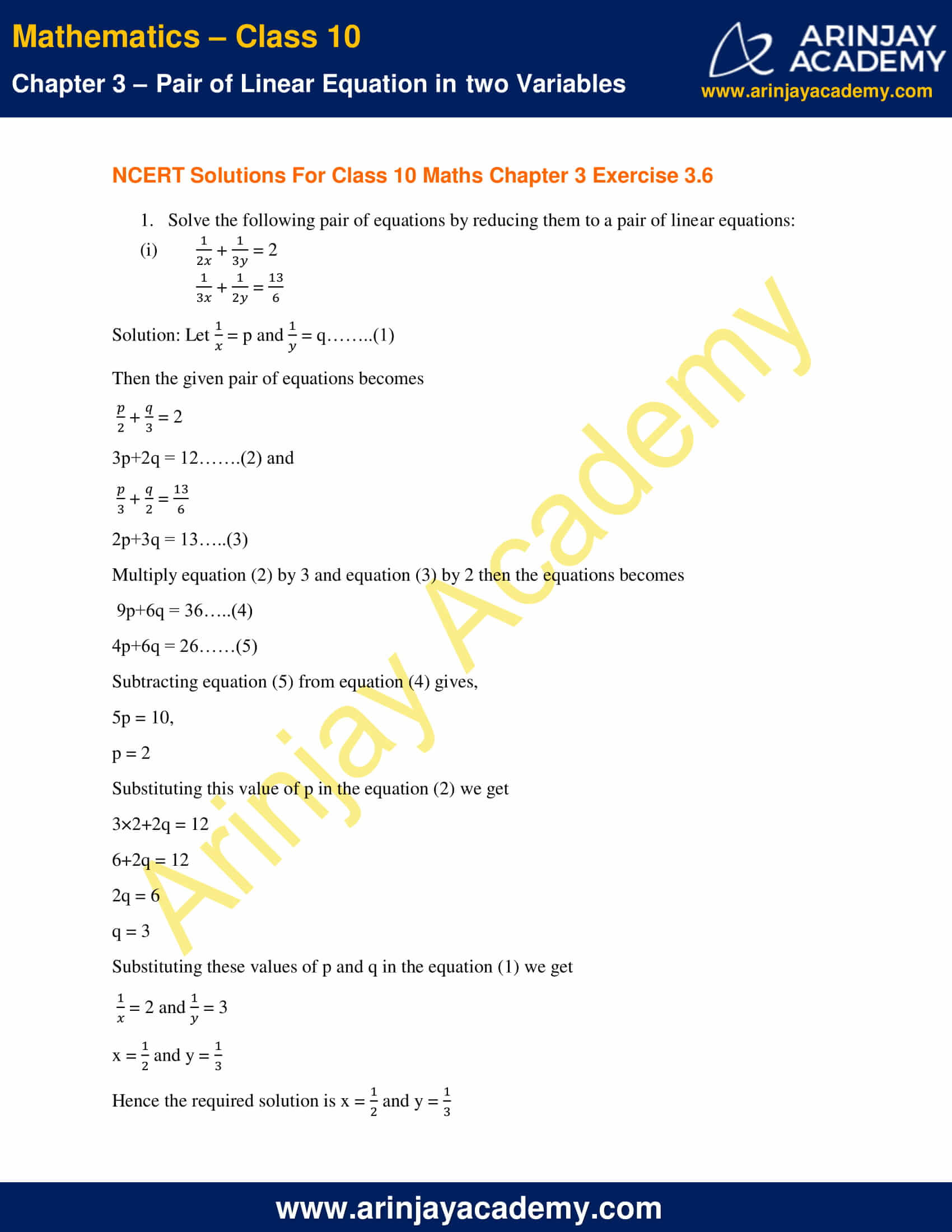
1. Solve the following pair of equations by reducing them to a pair of linear equations:

(i) $\cfrac { 1 }{ 2x }$ + $\cfrac { 1 }{ 3y }$ = 2

$\cfrac { 1 }{ 2x }$ + $\cfrac { 1 }{ 2y }$ = $\cfrac { 13 }{ 6 }$

Solution: Let $\cfrac { 1 }{ x }$ = p and $\cfrac { 1 }{ y }$ = q……..(1)

Then the given pair of equations becomes

$\cfrac { p }{ 2 }$ + $\cfrac { q }{ 3 }$ = 2

3p+2q = 12…….(2) and

$\cfrac { p }{ 3 }$ + $\cfrac { q }{ 2 }$ = $\cfrac { 13 }{ 6 }$

2p+3q = 13…..(3)

Multiply equation (2) by 3 and equation (3) by 2 then the equations becomes

9p+6q = 36…..(4)

4p+6q = 26……(5)

Subtracting equation (5) from equation (4) gives,

5p = 10,

p = 2

Substituting this value of p in the equation (2) we get

3×2+2q = 12

6+2q = 12

2q = 6

q = 3

Substituting these values of p and q in the equation (1) we get

$\cfrac { 1 }{ x }$ = 2 and $\cfrac { 1 }{ y }$ = 3

x = $\cfrac { 1 }{ 2 }$ and y = $\cfrac { 1 }{ 3 }$

Hence the required solution is x = $\cfrac { 1 }{ 2 }$ and y = $\cfrac { 1 }{ 3 }$

(ii) $\cfrac { 2 }{ \sqrt { x } }$ + $\cfrac { 3 }{ \sqrt { y } }$ = 2

$\cfrac { 4 }{ \sqrt { x } }$ – $\cfrac { 9 }{ \sqrt { y } }$ = -1

Solution: Let $\cfrac { 1 }{ \sqrt { x } }$ = p and $\cfrac { 1 }{ \sqrt { y } }$ = q……..(1)

Then the given pair of equations becomes

2p+3q = 2…..(2) and

4p-9q = -1……(3)

Multiply equation (2) by 3 and equation (3) by 1 then the equations becomes

6p+9q = 6…..(4)

4p-9q = -1……(5)

Adding equation (4) and equation (5) gives,

10p = 5

p = $\cfrac { 1 }{ 2 }$

Substituting this value of p in the equation (2) we get

2× $\cfrac { 1 }{ 2 }$ +3q = 2

3q =2-1

3q = 1

q = $\cfrac { 1 }{ 3 }$

Substituting these values of p and q in the equation (1) we get

$\cfrac { 1 }{ \sqrt { x } }$ = $\cfrac { 1 }{ 2 }$ and $\cfrac { 1 }{ \sqrt { y } }$ = $\cfrac { 1 }{ 3 }$

√x = 2 and √y = 3

x = 4 and y = 9

Hence the required solution is x = 4 and y = 9

(iii) $\cfrac { 4 }{ x }$ +3y = 14

$\cfrac { 3 }{ x }$ -4y = 23

Solution: Let $\cfrac { 1 }{ x }$ = p…..(1)

Then the given equation reduces to

4p+3y = 14……(2) and

3p-4y = 23…….(3)

Multiply the equation (2) by 4 and equation (3) by 3 we have

16p+12y = 56……(4) and

9p-12y = 69………(5)

Adding the equations (4) and (5) we get

25p = 125

p = 5

Substituting the value of p in the equation (2)

4×5+3y = 14

20+3y = 14

3y = -6

y = -2

Then from equation (1) we get,

$\cfrac { 1 }{ x }$ = 5

x = $\cfrac { 1 }{ 5 }$

Hence the required solution is x = $\cfrac { 1 }{ 5 }$ and y = -2

(iv) $\cfrac { 5 }{ x-1 }$ + $\cfrac { 1 }{ y-2 }$ = 2

$\cfrac { 6 }{ x-1 }$ – $\cfrac { 3 }{ y-2 }$ = 1

Solution: Let $\cfrac { 1 }{ x-1 }$ = p and $\cfrac { 1 }{ y-2 }$ = q…..(1)

Then the given pair of equation reduces to

5p+q = 2…..(2) and

6p-3q = 1…..(3)

Multiply the equation (2) by 3 we get

15p+3q = 6…..(4)

Adding the equations (3) and (4)

21p = 7

p = $\cfrac { 1 }{ 3 }$

Substituting the value of p in the equation (2)

5× $\cfrac { 1 }{ 3 }$ +q = 2

q = 2- $\cfrac { 5 }{ 3 }$

q = $\cfrac { 1 }{ 3 }$

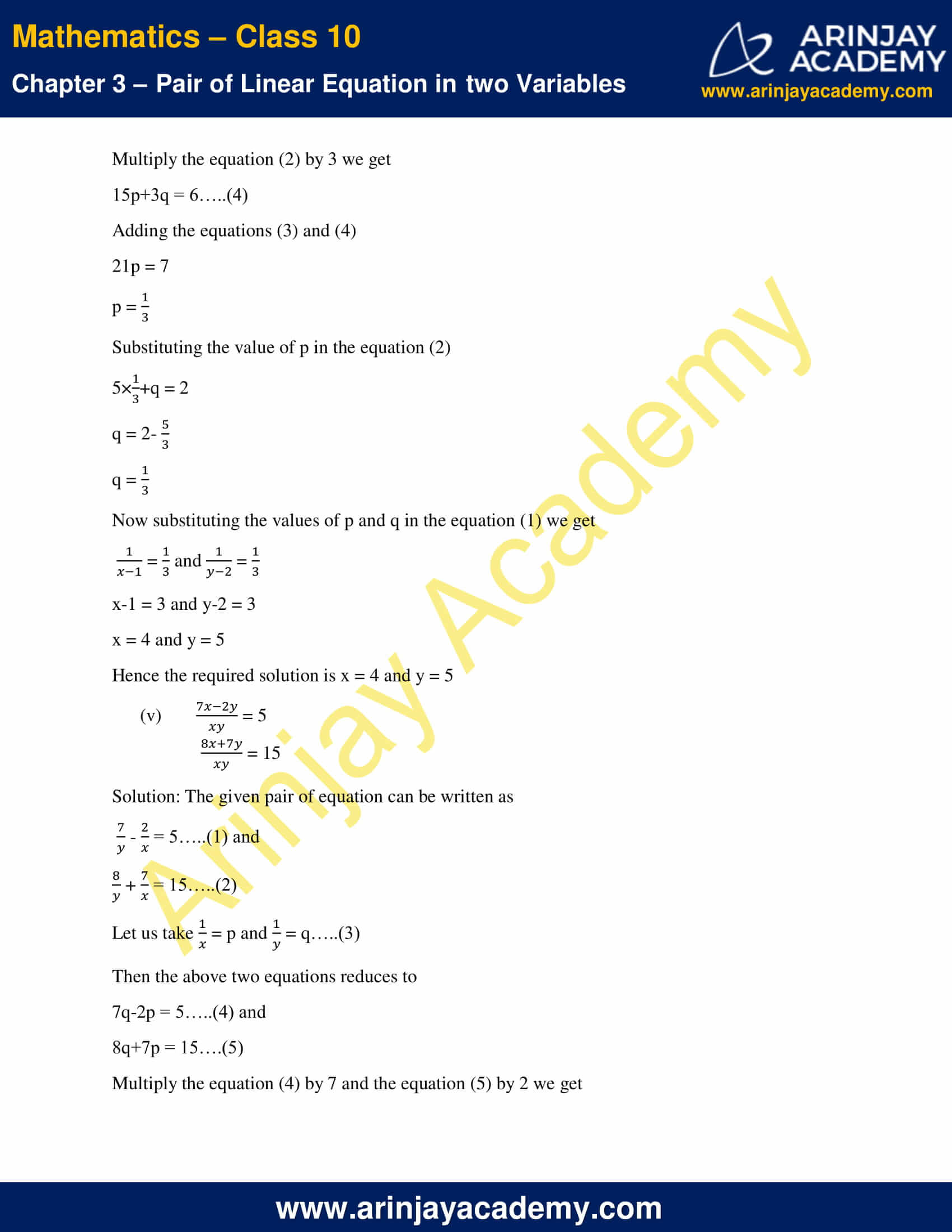
Now substituting the values of p and q in the equation (1) we get

$\cfrac { 1 }{ x-1 }$ = $\cfrac { 1 }{ 3 }$ and $\cfrac { 1 }{ y-2 }$ = $\cfrac { 1 }{ 3 }$

x-1 = 3 and y-2 = 3

x = 4 and y = 5

Hence the required solution is x = 4 and y = 5

(v) $\cfrac { 7x-2y }{ xy }$ = 5

$\cfrac { 8x+7y }{ xy }$ = 15

Solution: The given pair of equation can be written as

$\cfrac { 7 }{ y }$ – $\cfrac { 2 }{ x }$ = 5…..(1) and

$\cfrac { 8 }{ y }$ + $\cfrac { 7 }{ x }$ = 15…..(2)

Let us take $\cfrac { 1 }{ x }$ = p and $\cfrac { 1 }{ y }$ = q…..(3)

Then the above two equations reduces to

7q-2p = 5…..(4) and

8q+7p = 15….(5)

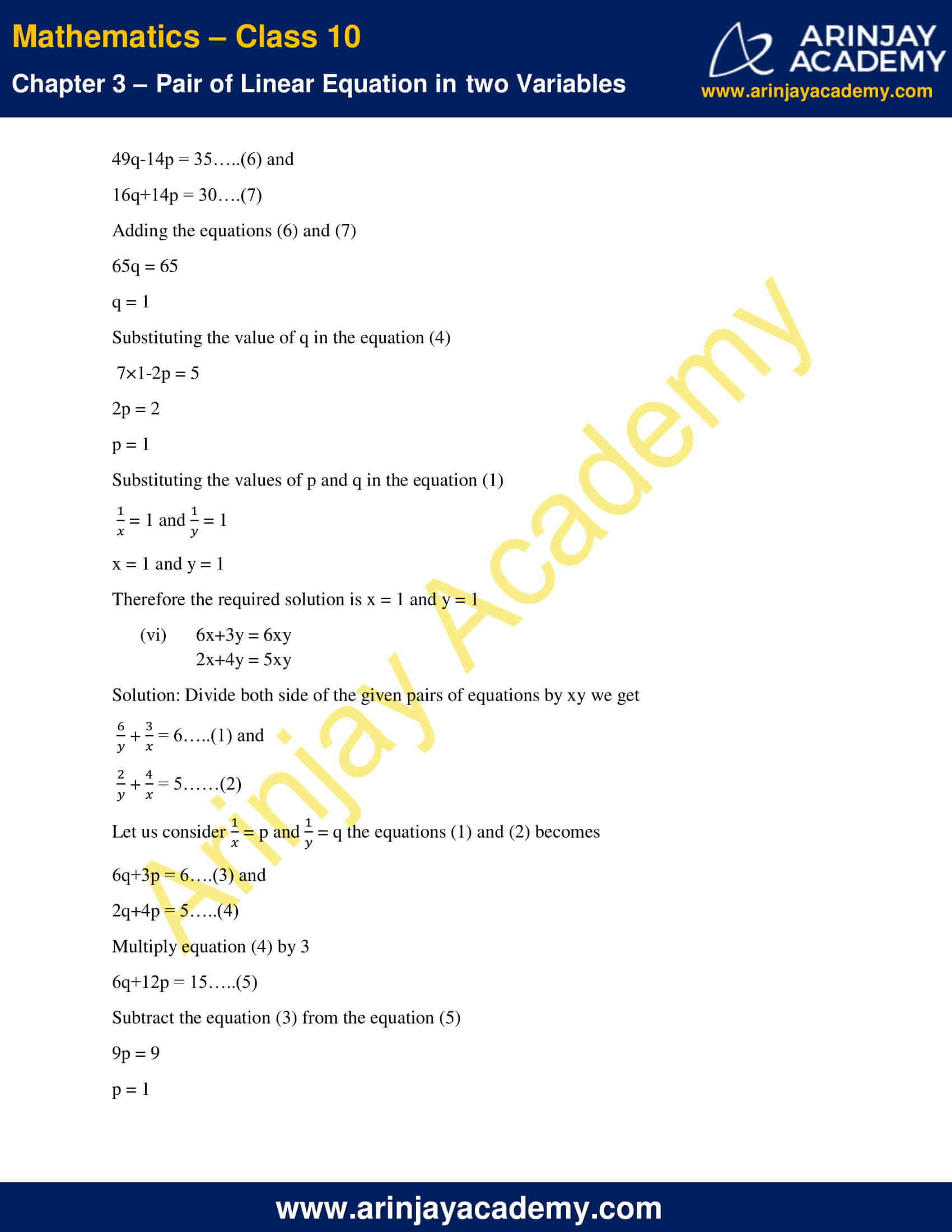
Multiply the equation (4) by 7 and the equation (5) by 2 we get

49q-14p = 35…..(6) and

16q+14p = 30….(7)

Adding the equations (6) and (7)

65q = 65

q = 1

Substituting the value of q in the equation (4)

7×1-2p = 5

2p = 2

p = 1

Substituting the values of p and q in the equation (1)

$\cfrac { 1 }{ x }$ = 1 and $\cfrac { 1 }{ y }$ = 1

x = 1 and y = 1

Therefore the required solution is x = 1 and y = 1

(vi) 6x+3y = 6xy

2x+4y = 5xy

Solution: Divide both side of the given pairs of equations by xy we get

$\cfrac { 6 }{ y }$ + $\cfrac { 3 }{ x }$ = 6…..(1) and

$\cfrac { 2 }{ y }$ + $\cfrac { 4 }{ x }$ = 5……(2)

Let us consider $\cfrac { 1 }{ x }$ = p and $\cfrac { 1 }{ y }$ = q the equations (1) and (2) becomes

6q+3p = 6….(3) and

2q+4p = 5…..(4)

Multiply equation (4) by 3

6q+12p = 15…..(5)

Subtract the equation (3) from the equation (5)

9p = 9

p = 1

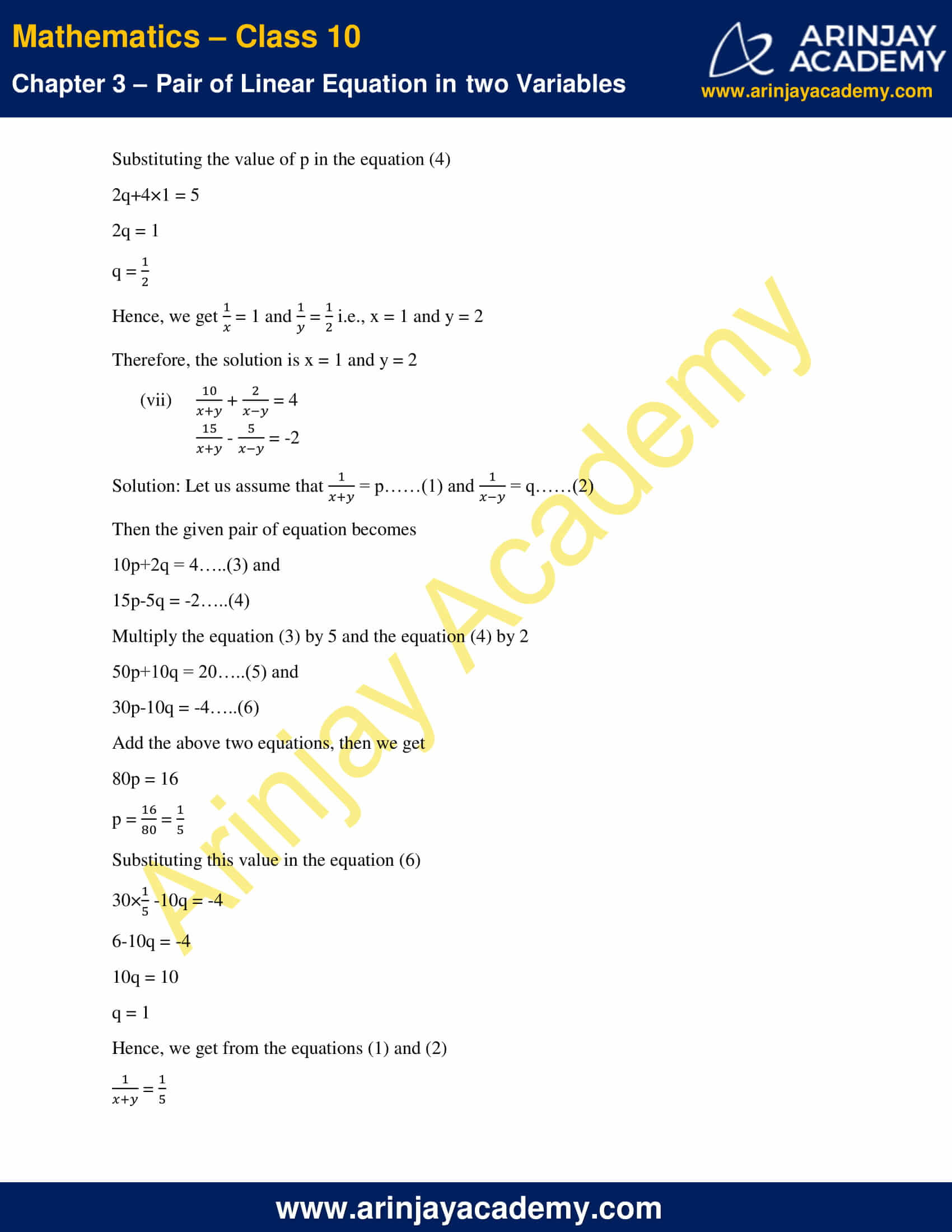
Substituting the value of p in the equation (4)

2q+4×1 = 5

2q = 1

q = $\cfrac { 1 }{ 2 }$

Hence, we get $\cfrac { 1 }{ x }$ = 1 and $\cfrac { 1 }{ y }$ = $\cfrac { 1 }{ 2 }$ i.e., x = 1 and y = 2

Therefore, the solution is x = 1 and y = 2

(vii) $\cfrac { 10 }{ x+y }$ + $\cfrac { 2 }{ x-y }$ = 4

$\cfrac { 15 }{ x+y }$ – $\cfrac { 5 }{ x-y }$ = -2

Solution: Let us assume that $\cfrac { 1 }{ x+y }$ = p……(1) and $\cfrac { 1 }{ x-y }$ = q……(2)

Then the given pair of equation becomes

10p+2q = 4…..(3) and

15p-5q = -2…..(4)

Multiply the equation (3) by 5 and the equation (4) by 2

50p+10q = 20…..(5) and

30p-10q = -4…..(6)

Add the above two equations, then we get

80p = 16

p = $\cfrac { 16 }{ 80 }$ = $\cfrac { 1 }{ 5 }$

Substituting this value in the equation (6)

30× $\cfrac { 1 }{ 5 }$ -10q = -4

6-10q = -4

10q = 10

q = 1

Hence, we get from the equations (1) and (2)

$\cfrac { 1 }{ x+y }$ = $\cfrac { 1 }{ 5 }$

x+y = 5……(7)and

$\cfrac { 1 }{ x-y }$ = 1

x-y = 1….(8)

Adding the equation (7) and (8) we get

2x = 6

x = 3

And substituting the value x = 3 in the equation (7)

3+y = 5

y = 2

Therefore the required solution is x = 3 and y = 2

(viii) $\cfrac { 1 }{ 3x+4 }$ + $\cfrac { 1 }{ 3x-y }$ = $\cfrac { 3 }{ 4 }$

$\cfrac { 1 }{ 2(3x+y) }$ – $\cfrac { 1 }{ 2(3x-y) }$ = – $\cfrac { 1 }{ 8 }$

Solution: Let us consider that

$\cfrac { 1 }{ 3x+y }$ = p…..(1) and $\cfrac { 1 }{ 3x-y }$ = q…..(2)

Then the given pair of equations becomes,

p+q = $\cfrac { 3 }{ 4 }$

4p+4q = 3…..(3) and

$\cfrac { p }{ 2 }$ – $\cfrac { q }{ 2 }$ = $\cfrac { -1 }{ 8 }$

4p-4q = -1…..(4)

Adding the equations (3) and (4) we get

8p = 2

p = $\cfrac { 1 }{ 4 }$

Substituting the value of p in the equation (3)

4× $\cfrac { 1 }{ 4 }$ +4q = 3

1+4q = 3

4q = 2

q = $\cfrac { 1 }{ 2 }$

Now substituting the values of p and q in the equation (1) and (2) respectively we have

$\cfrac { 1 }{ 3x+y }$ = $\cfrac { 1 }{ 4 }$

3x+y = 4…..(5) and

$\cfrac { 1 }{ 3x-y }$ = $\cfrac { 1 }{ 2 }$

3x-y = 2…..(6)

Adding the equations (5) and (6)

6x = 6

x = 1

And substituting the value of x in the equation (5) we have

3×1+y = 4

3+y = 4

y = 1

Therefore, the required solution is x = 1 and y = 1

2. Formulate the following problems as a pair of equations and hence find their solutions:

(i) Ritu can row downstream 20 km in 2 hours and upstream 4 km in 2 hours. Find her speed of rowing in still water and the speed of the current.

Solution: Let the speed of rowing of Ritu in still water is x km/hr and the speed of current is y km/hr.

Speed in downstream is x+y km/hr and Speed in upstream is x-y km/hr.

Speed = $\cfrac { Distance }{ Time }$

Then using the given conditions, we have the equations as

$\cfrac { 20 }{ x+y }$ = 2

x+y = 10….(1)

$\cfrac { 4 }{ x-y }$ = 2

x-y = 2….(2)

Adding the equations (1) and (2) we get,

2x = 12

x = 6

Substituting the value of x in the equation (1) we get

6+y = 10

y = 4

Therefore, the speed of rowing of Ritu in still water is 6 km/hr and the speed of current is 4 km/hr.

(ii) 2 women and 5 men can together finish an embroidery work in 4 days, while 3 women and 6 men can finish it in 3 days. Find the time taken by 1 woman alone to finish the work, and also that taken by 1 man alone.

Solution: Let x be the time taken by 1 woman alone to finish the work and y be the time taken by 1 man alone. And let the whole work is 1

1 woman 1-day work = $\cfrac { 1 }{ x }$

1 man one day work = $\cfrac { 1 }{ y }$

Then using given condition we get the equations as

4( $\cfrac { 2 }{ x }$ + $\cfrac { 5 }{ y }$ ) = 1….(1)and

3( $\cfrac { 3 }{ x }$ + $\cfrac { 6 }{ y }$ ) = 1…..(2)

Now let us consider

$\cfrac { 1 }{ x }$ = p and $\cfrac { 1 }{ y }$ = q……(3)

Then the equations (1) and (2) reduces to

4(2p+5q) = 1

8p+20q = 1……(4) and

3(3p+6q) = 1

9p+18q = 1……(5)

Multiply the equation (4) by 9 and the equation (5) by 8 we have

72p+180q = 9….(6) and

72p+144q = 8……(7)

Subtracting equation (7) from equation (6)

36q = 1

q = $\cfrac { 1 }{ 36 }$

And substituting this value of p in the equation (5) we get

9p+18× $\cfrac { 1 }{ 36 }$ = 1

9p = 1- $\cfrac { 1 }{ 2 }$

9p = $\cfrac { 1 }{ 2 }$

p = $\cfrac { 1 }{ 18 }$

Substituting this value of p and q in the equation (iii) we get

$\cfrac { 1 }{ x }$ = $\cfrac { 1 }{ 18 }$ and $\cfrac { 1 }{ y }$ = $\cfrac { 1 }{ 36 }$

x = 18 and y = 36

Therefore, the time taken by 1 woman alone to finish the work is 18 days and the time taken by a man to finish the work alone is 36 days.

(iii) Roohi travels 300 km to her home partly by train and partly by bus. She takes 4 hours if she travels 60 km by train and the remaining by bus. If she travels 100 km by train and the remaining by bus she takes 10 min longer. Find the speed of the train and the bus separately.

Solution: Let the speed of the train is x km/hr and speed of bus is y km/hr.

She takes 4 hr when she travel 60 km by train and (300-60) = 240 km by bus and takes 10 minutes longer when she travels 100 km by train and (300-100) = 200 km by bus.

Also 10 minutes = $\cfrac { 10 }{ 60 }$ = $\cfrac { 1 }{ 6 }$ hours

Since we know that, Time = $\cfrac { Distance }{ Speed }$ then using the given conditions we have

$\cfrac { 60 }{ x }$ + $\cfrac { 240 }{ y }$ = 4

$\cfrac { 15 }{ x }$ + $\cfrac { 60 }{ y }$ = 1…..(1) and

$\cfrac { 100 }{ x }$ + $\cfrac { 200 }{ y }$ = 4+ $\cfrac { 1 }{ 6 }$

$\cfrac { 100 }{ x }$ + $\cfrac { 200 }{ y }$ = $\cfrac { 25 }{ 6 }$

$\cfrac { 4 }{ x }$ + $\cfrac { 8 }{ y }$ = $\cfrac { 1 }{ 6 }$ …….(2)

Let us assume that $\cfrac { 1 }{ x }$ = p and $\cfrac { 1 }{ y }$ = q then the above two equations reduces to,

15p+60q = 1

p = $\cfrac { 1-60q }{ 15 }$ …..(3) and

4p+8q = $\cfrac { 1 }{ 6 }$

24p+48q = 1…..(4)

Substitute the value of p from the equation (3) in the equation (4) we get

24. $\cfrac { 1-60q }{ 15 }$ +48q = 1

8. $\cfrac { 1-60q }{ 5 }$ +48q = 1

8-480q+240q = 5

-240q = -3

q = $\cfrac { -3 }{ -240 }$

q = $\cfrac { 1 }{ 80 }$

Substitute this value of q in the equation (3)

p = $\cfrac { 1-60\times \cfrac { 1 }{ 80 } }{ 15 }$

= $\cfrac { 1-\cfrac { 3 }{ 4 } }{ 15 }$

= $\cfrac { 1 }{ 60 }$

Therefore, we have

$\cfrac { 1 }{ x }$ = $\cfrac { 1 }{ 60 }$ and $\cfrac { 1 }{ y }$ = $\cfrac { 1 }{ 80 }$

x = 60 and y = 80

Hence speed of the train is 60 km/ hr and that of the bus is 80 km/hr.

NCERT Solutions for Class 10 Maths Chapter 3 Exercise 3.6 – Pair of Linear Equations in two Variables, has been designed by the NCERT to test the knowledge of the student on the topic – Equations Reducible to a Pair of Linear Equations in Two Variables

Download NCERT Solutions For Class 10 Maths Chapter 3 Exercise 3.6 – Pair of Linear Equations in two Variables

NCERT Solutions For Class 10 Maths Chapter 3 Exercise 3.6 – Pair of Linear Equations in two Variables

NCERT Solutions For Class 10 Maths Chapter 3 Exercise 3.6 – Pair of Linear Equations in two Variables

NCERT Solutions for Class 10

CBSE Notes for Class 10

MCQ Questions for Class 10

Leave a Comment Cancel reply