NCERT Solutions For Class 10 Maths Chapter 11 Exercise 11.1 Constructions

NCERT Solutions For Class 10 Maths Chapter 11 Exercise 11.1 Constructions

NCERT Solutions For Class 10 Maths Chapter 11 Exercise 11.1 Constructions. This class 10th math ex. 11.1 contains 7 questions, for which detailed answers have been provided in this note. In case you are looking at studying the remaining Exercise for Class 10 for Maths NCERT solutions for other Chapters, you can click the link at the end of this Note.

Category	NCERT Solutions for Class 10
Subject	Maths
Chapter	Chapter 11
Exercise	Exercise 11.1
Chapter Name	Constructions

NCERT Solutions For Class 10 Maths Chapter 11 Exercise 11.1 Constructions

In each of the following, give the justification of the construction also:

1. Draw a line segment of length 7.6 cm and divide it in the ratio 5:8. Measure the two parts.

Solution:

A line segment AB of length 7.6 cm can be divided in the ratio 5:8 in following way:

 

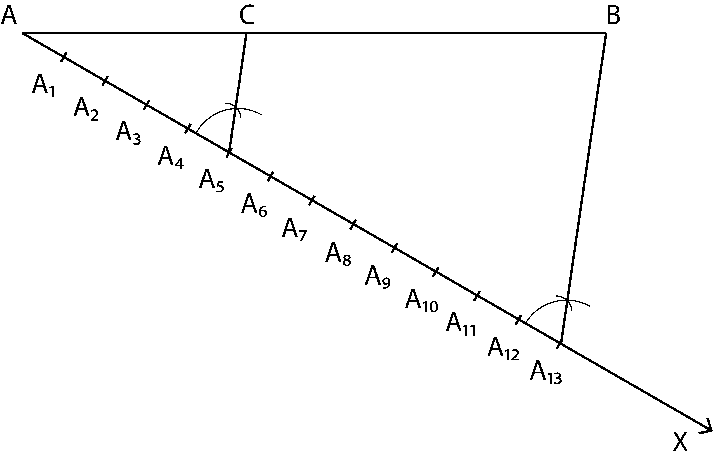
Steps of construction:

• Step 1. Draw any ray AX, making an acute angle with AB
• Step 2. Locate 13(= 5 + 8) points A₁, A₂, A₃, … , A₁₃ on AX so that AA₁ = A₁A₂ = A₂A₃ = … = A₁₂A₁₃
• Step 3. Join BA₁₃
• Step 4. Through the point A₅, draw a line parallel to A₁₃B(by making angle equal to ∠AA₁₃B) at A₅ intersecting AB at the point C

Then, AC : CB = 5 : 8
The length of AB and AC are 2.9 cm and 4.7 cm respectively.

Justification
Since A₅C is parallel to A₁₃B
∴ = (By the Basic Proportionality Theorem)

By construction, =
∴ =

This shows that C divides AB in the ratio 5 : 8.

2. Construct a triangle of sides 4 cm, 5 cm and 6 cm and then a triangle similar to it whose sides are of the corresponding sides of the first triangle.

Solution:

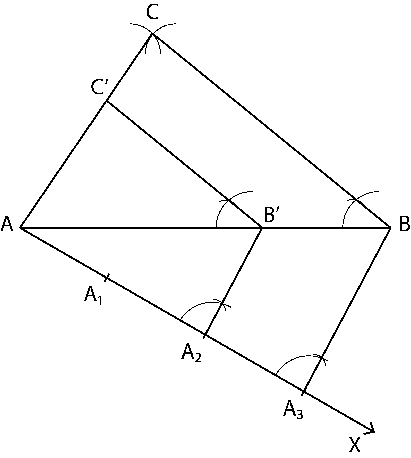
Steps of construction

• Step 1 – Draw a line segment AB = 4 cm
• Step 2 – With A as centre and radius 5 cm, draw an arc on one side of AB
• Step 3 – With B as centre and radius 6 cm, draw an arc intersecting the previous arc at C
• Step 4 – Join AC and BC
• Step 5 – Draw , making an acute angle with AB on opposite side of C
• Step 6 – Locate 3 points A₁, A₂ and A₃ on , such that AA₁ = A₁A₂ = A₂A₃
• Step 7 – Join BA₃
• Step 8 – Through A₂, draw a line parallel to BA₃, intersecting AB at B’
• Step 9 – Through B’, draw a line parallel to BC, intersecting AC at C’

The sides of △AB’C’ are times that of △ABC.

Justification

In △AB’A₂ and △ABA₃
∠A₂AB’ = ∠A₃AB  (Common)
∠AA₂B’ = ∠AA₃B   (Corresponding angles)
∴ △AB’A₂ ∼ △ABA₃ (AA similarity criteria)
⇒ = =     …(i)
In △AB’C’ and △ABC
∠C’AB’ = ∠CAB  (Common)
∠AB’C’ = ∠ABC  (Corresponding angles)
∴ △AB’C’ ∼ △ABC
⇒ = =  …(ii)
From equation (i) and (ii)
= = =
Therefore, sides of △AB’C’ are  times that of △ABC.

NCERT Solutions for Class 10 Maths Chapter 11 Exercise 11.1

3. Construct a triangle with sides 5 cm, 6 cm and 7 cm and then another triangle whose sides are of the corresponding sides of the first triangle.

Solution:

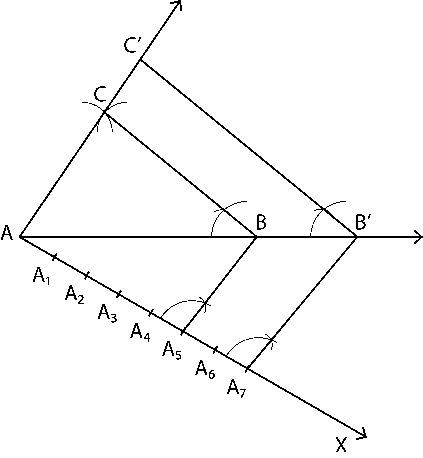
Steps of construction

• Step 1 – Draw a line segment AB = 5 cm
• Step 2 – With A as centre and radius 6 cm, draw an arc on one side of AB
• Step 3 – With B as centre and radius 7 cm, draw an arc intersecting the previous arc at C
• Step 4 – Join AC and BC
• Step 5 – Draw , making an acute angle with AB on opposite side of C
• Step 6 – Locate 7 points A₁, A₂,… , A₇ on , such that AA₁ = A₁A₂ = … = A­₆A₇
• Step 7 – Join BA₅
• Step 8 – Through A₇, draw a line parallel to BA₅, intersecting AB produced at B’
• Step 9 – Through B’, draw a line parallel to BC, intersecting AC produced at C’

The sides of △AB’C’ are times that of △ABC.

Justification

In △AB’A₇ and △ABA₅
∠A₇AB’ = ∠A₅AB  (Common)
∠AA₇B’ = ∠AA₅B   (Corresponding angles)
∴ △AB’A₇ ∼ △ABA₅ (AA similarity criteria)
⇒ = =    …(i)

In △AB’C’ and △ABC
∠C’AB’ = ∠CAB  (Common)
∠AB’C’ = ∠ABC  (Corresponding angles)
∴ △AB’C’ ∼ △ABC
⇒ = = …(ii)

From equation (i) and (ii)
= = =
Therefore, sides of △AB’C’ are times that of △ABC.

4. Construct an isosceles triangle whose base is 8 cm and altitude 4 cm and then another triangle whose sides are times the corresponding sides of the isosceles triangle.

Solution:

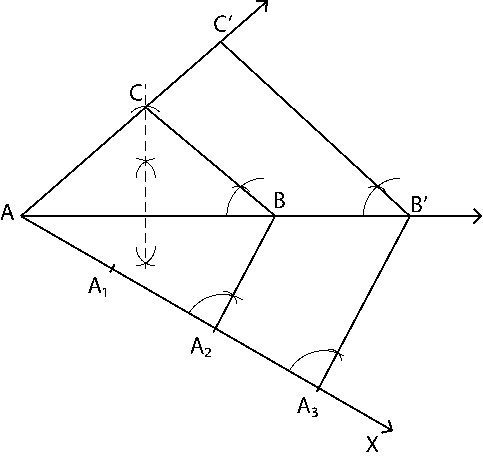
Steps of construction

• Step 1 – Draw a line segment AB = 8 cm
• Step 2 – Draw a perpendicular bisector of AB
• Step 3 – Mark D as the mid-point of AB and C on the perpendicular bisector at 4 cm from D
• Step 4 – Join AC and BC
• Step 5 – Draw , making an acute angle with AB on opposite side of C
• Step 6 – Locate 3 points A₁, A₂, A₃ on , such that AA₁ = A₁A₂ = A­₂A₃
• Step 7 – Join BA₂
• Step 8 – Through A₃, draw a line parallel to BA₂, intersecting AB produced at B’
• Step 9 – Through B’, draw a line parallel to BC, intersecting AC produced at C’

The sides of △AB’C’ are = times that of △ABC.

Justification

In △AB’A₃ and △ABA₂
∠A₃AB’ = ∠A₂AB   (Common)
∠AA₃B’ = ∠AA₂B    (Corresponding angles)
∴ △AB’A₃ ∼ △ABA₂ (AA similarity criteria)
⇒ = =     …(i)

In △AB’C’ and △ABC
∠C’AB’ = ∠CAB  (Common)
∠AB’C’ = ∠ABC  (Corresponding angles)
∴ △AB’C’ ∼ △ABC
⇒ = =    …(ii)

From equation (i) and (ii)
= = = =
Therefore, sides of △AB’C’ are  times that of △ABC.

Class 10th Maths 11.1 NCERT Solutions

5. Draw a triangle ABC with side BC = 6 cm, AB = 5 cm and ∠ABC = 60°. Then construct a triangle whose sides are of the corresponding sides of the triangle ABC.

Solution:

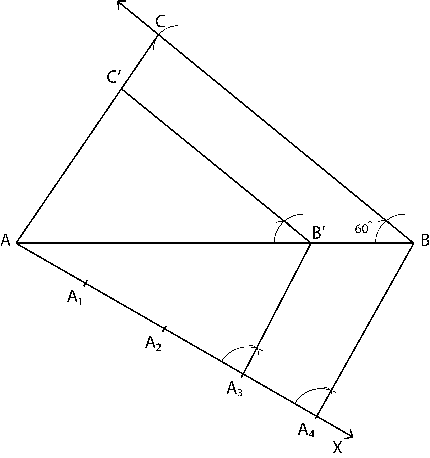
Steps of construction

• Step 1 – Draw a line segment AB = 5 cm
• Step 2 – Make ∠CBA = 60° such that BC = 6 cm
• Step 3 – Join AC
• Step 4 – Draw , making an acute angle with AB on opposite side of C
• Step 5 – Locate 7 points A₁, A₂,… , A₄ on , such that AA₁ = A₁A₂ = … = A­₃A₄
• Step 6 – Join BA₄
• Step 7 – Through A₃, draw a line parallel to BA₄, intersecting AB produced at B’
• Step 8 – Through B’, draw a line parallel to BC, intersecting AC produced at C’

The sides of △AB’C’ are times that of △ABC.

Justification

In △AB’A₃ and △ABA₄
∠A₃AB’ = ∠A₄AB   (Common)
∠AA₃B’ = ∠AA₄B   (Corresponding angles)
∴ △AB’A₃ ∼ △ABA₄ (AA similarity criteria)
⇒ = =  …(i)

In △AB’C’ and △ABC
∠C’AB’ = ∠CAB (Common)
∠AB’C’ = ∠ABC (Corresponding angles)
∴ △AB’C’ ∼ △ABC
⇒ = =  …(ii)

From equation (i) and (ii)
= = =
Therefore, sides of △AB’C’ are  times that of △ABC.

6. Draw a triangle ABC with side BC = 7 cm, ∠ B = 45°, ∠ A = 105°. Then, construct a triangle whose sides are times the corresponding sides of △ABC.

Solution:

In △ABC
∠ A + ∠ B + ∠ C = 180°
105° + 45° + ∠ C = 180°
150° + ∠ C = 180°
∠ C = 30°

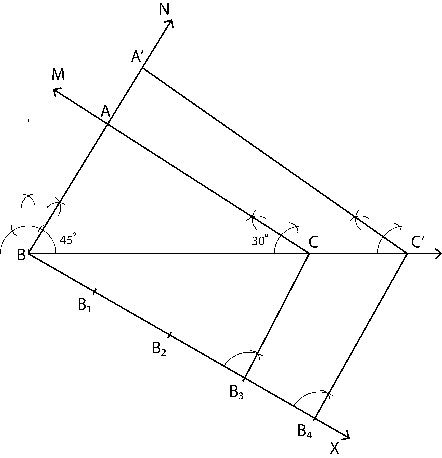
 Steps of construction

• Step 1 – Draw a line segment BC = 7 cm
• Step 2 – Make ∠CBN = 45° and ∠BCM = 30°
• Step 3 – Mark the point of intersection of BN and CM as A
• Step 4 – Draw , making an acute angle with BC on opposite side of A
• Step 5 – Locate 4 points B₁, B₂,… , B₄ on , such that BB₁ = B₁B₂ = … = B­₃B₄
• Step 6 – Join CB₃
• Step 7 – Through B₄, draw a line parallel to CB₃, intersecting BC produced at C’
• Step 8 – Through C’, draw a line parallel to AC, intersecting BA produced at A’

The sides of △A’BC’ are times that of △ABC.

Justification

In △BC’B₄ and △BCB₃
∠B₄BC’ = ∠B₃BC      (Common)
∠BB₄C’ = ∠BB₃C      (Corresponding angles)
∴ △BC’B₄ ∼ △BCB₃ (AA similarity criteria)
⇒  = = …(i)

In △A’BC’ and △ABC
∠A’BC’ = ∠ABC    (Common)
∠BC’A’ = ∠BCA    (Corresponding angles)
∴ △A’BC’ ∼ △ABC
⇒ = = …(ii)

From equation (i) and (ii)
= = =
Therefore, sides of △A’BC’ are  times that of △ABC.

NCERT Solutions for Class 10 Maths Chapter 11 Exercise 11.1

7. Draw a right triangle in which the sides (other than hypotenuse) are of lengths 4 cm and 3 cm. Then construct another triangle whose sides are times the corresponding sides of the given triangle.

Solution:

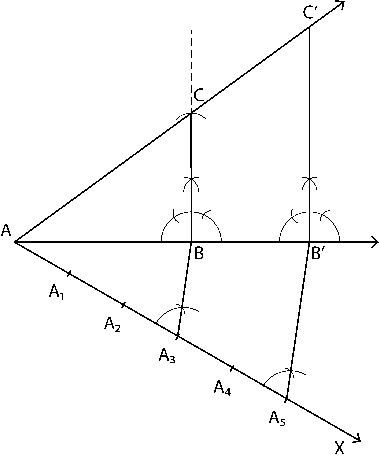
Steps of construction

• Step 1 – Draw a line segment AB = 4 cm
• Step 2 – Draw ∠CBA = 90°, such that BC = 3 cm
• Step 3 – Join AC
• Step 4 – Draw , making an acute angle with AB on opposite side of C
• Step 5 – Locate 5 points A₁, A₂,… , A₅ on , such that AA₁ = A₁A₂ = … = A­₄A₅
• Step 6 – Join BA₃
• Step 7 – Through A₅, draw a line parallel to BA₃, intersecting AB produced at B’
• Step 8 – Through B’, draw a line parallel to BC, intersecting AC produced at C’

The sides of △AB’C’ are times that of △ABC.

Justification

In △AB’A₅ and △ABA₃
∠A₅AB’ = ∠A₃AB   (Common)
∠AA₅B’ = ∠AA₃B   (Corresponding angles)
∴ △AB’A₅ ∼ △ABA₃ (AA similarity criteria)
⇒ = =  …(i)

In △AB’C’ and △ABC
∠C’AB’ = ∠CAB  (Common)
∠AB’C’ = ∠ABC   (Corresponding angles)
∴ △AB’C’ ∼ △ABC
⇒ = =   …(ii)

From equation (i) and (ii)
= = =
Therefore, sides of △AB’C’ are times that of △ABC.

NCERT Solutions for Class 10 Maths Chapter 11 Exercise 11.1 Constructions, has been designed by the NCERT to test the knowledge of the student on the topic – Division of a Line Segment

• NCERT Solutions Class 10 Maths Chapter 11 Ex. 11.1 Introduction to Trigonometry
• NCERT Solutions Class 10 Maths Chapter 11 Ex. 11.2 Introduction to Trigonometry
• Maths – NCERT Solutions Class 10
• NCERT Solutions Class 10

Download NCERT Solutions For Class 10 Maths Chapter 11 Exercise 11.1 Constructions