Comparing Rational Numbers – To compare any two Rational Number we have to follow the following steps:-
Step I: Express both the Rational numbers with positive Denominator.
Step II: Take the LCM of the positive Denominators.
Step III: Express both the Rational number obtained in step I with this LCM.
Step IV: The number with higher Numerator is greater.
Comparing Rational Numbers Example 1
Fill the missing place:
12/13 ___ 10/13
Explanation
1st number = 12/13
2nd number = 10/13
If two rational numbers have common denominators, the number with higher Numerator is greater
If we compare their Numerators, we notice that
12 > 10
Accordingly 12/13 > 10/13
Hence, 12/13 > 10/13
Comparing Rational Numbers Example 2
Fill the missing place:
(-3)/11 ___ 8/(-11)
Explanation
If we express both the Rational numbers with positive Denominator, we would get
1st number = (-3)/11
2nd number = 8/(-11)
To express 8/(-11) with a positive Denominator we would multiply both its Numerator and Denominator by (-1).
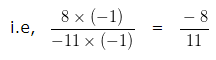
If two rational numbers have common denominators, the number with higher Numerator is greater
If we compare their Numerators, we notice that
-3 > -8
then, (-3)/11 > (-8)/11
Hence, (-3)/11 > 8/(-11)
Comparing Rational Numbers Example 3
Fill the missing place:
(-2)/5 ___ 5/(-4)
Explanation
If we express both the Rational numbers with positive Denominator, we would get
1st number = (-2)/5
2nd number = 5/(-4)
To express 5/(-4) with a positive Denominator we would multiply both its Numerator and Denominator by (-1).
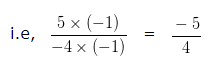
Since Denominators are different, we would need to solve the given equations in the following manner
Take LCM of 5 and 4 which is 20
Multiply both the Numerator and Denominator of (-2)/5 by 4 we would get .
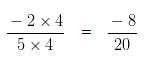
Similarly, Multiplying Numerator and Denominator of (-5)/4 by 5 .
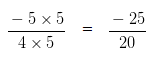
If two rational numbers have common denominators, the number with higher Numerator is greater
On comparing Numerators of (-8)/20 and (-25)/20
we find -8 > -25
then, (-8)/20 > (-25)/20
Hence, (-2)/5 > 5/(-4)